1at pi / 2 , sin x is 1 ( seems you dozed off ) anyway , answer is 3
Find the no of solutions.
2cosx=\left|sinx \right|x belongs to 0 to 2pie
-
UP 0 DOWN 0 0 22
22 Answers
11if two curves are symmetric abt any fixed line doesn't make any thing easier -- you can't just say what you said -- tell you what , this came in FIITJEE and they also solved it wrongly
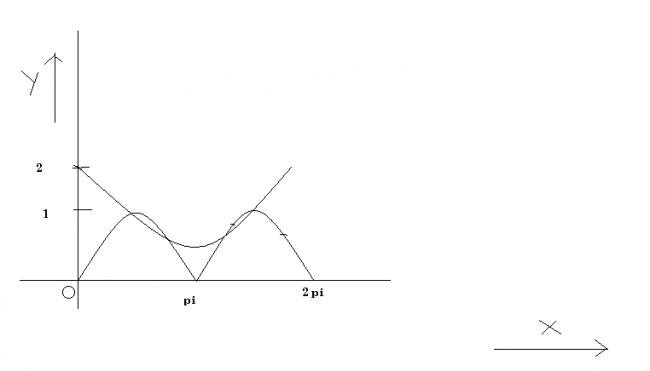
1As everyone knows...the 2 bumps r symmetric about x = pi.
And the graph of 2^cos x is also symmetric about x = pi.
So wht u've predicted is merely a wrong assumption.
And soumya stop thinking abt. this ... the Joker has already said "why so serious ??"
1but once the graph of 2^cos x cuts that of | sin x | , the following things happen --
as | sin x | and cos x are both increasing in the interval pi - 3 pi / 2 , so obviously the graph of 2 ^ cos x will rise more sharply than that of the sine one -- so I don't think there will be 4 solutions ---
maybe I really am wrong -- I welcome everyone to clear this mess up -- : ) : 0
1Once again I say.it's 4 solutions...........isn't it Joker ???
1so is the DARK KNIGHT not once again teaming up with the JOKER ???? the answer is 4 indeed.
if you have doudts tell at the chat box
1I really dozed off..........like years of rivalry once again the DARK KNIGHT and the JOKER differ in their viewpoints ....... the answer is 3 indeed.....now it's too tedious to post the graphs....but i've done it ... kk thnx dude...'twas a good sum. neways...2^cos x graph was right na ??
6its easy ...nishant bhaiya u gave d question to soumya...n he gave me....
1ARE YOU MAD ???????????????? HOW CAN | sin x | HAVE A VALUE 0 AT pi / 2 ????????????????
1This is the first time ever that the Joker and the Dark Knight have shared a common idea .
1Clearly der r 4 solns. .
the graph of 2^cos x cuts the sin curve in the middle 2 bumps at 4 points.
1hey kalyan , seems no body is interested ---- draw the graphs folks