I am sorry, could you clarify whether my solution is right or wrong?
I tore several successive pages out of a book. The number of the first page I tore was 183 while the number of the last page was written with the same digits in some order. How many pages did I tear out of the book?
-
UP 0 DOWN 0 0 19

19 Answers
first we observe that in every book, we have a page numbered with an odd integer on the right hand side and with an even integer on the left hand side.
continuing this we know every time we tear one page, we are actually tearing 2 sides. eg if we tear pg 183, page 184 also comes along with that.
so we know every time we tear a page numbered 2n we also end up tearing (2n-1)th page. so the last side of a page we are tearing is always even.
so only possibility we have is the last page being 318.
so the the number of pages torn by us is (318-183 +1)/2 = 68 pages or 136 sides
ohhh... actually, i dunno d full form of WLOG... so got confused... [6] [4]
[1]
It could be. You would only be swapping d for a and c for b. The inequality would be unchanged. Hence I can assume Without Loss Of Generality that a<b<c<d
generally no buk starts wid page no. 1 on left side... [u must assume such general things while solving a problem] [4]
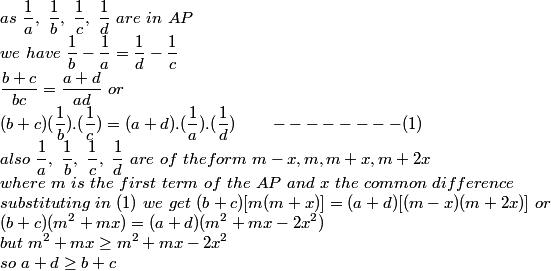
WLOG a<b<c<d
then 1/a > 1/b > 1/c > 1/d
Since they are in AP 1/a - 1/b = 1/c - 1/d,
so, b-a/ab = d-c/dc or b-a = (d-c) X (ab/dc) ≤ d-c
From this we get a+d ≥ b+c
because every buk starts wid page no. 1 on right side... so u can't start tearing pages from an even no. ........... [1]
hey subash...
u even can't start wid an even no. and end wid an odd no. ... u can only start wid an odd no. and end wid an even no. ... dats d only possibility...
because when u tear a set of consecutive papers
u hav to start with a page having even number and end with odd number or vice versa
otherwise u cant tear just one side of a paper
Abhirup, why is it that the last page no. must be an even one?
correct ans. that was too simple. wasnt it. here's another one
if positeve numbers a,b,c,d are such that 1/a , 1/b , 1/c , 1/d are in AP then show that a+d >= b+c
because the last page no. must be an even one....hence 318