13is it for powerplay or is it only for powerplay...!!! [6] [4]
12 Answers
106Ans is (-infinity, -1)U(-1/2,0)U(1/2, infinity)
is this correct??
13u haven't considered all cases rahul... think over ur solution once again, u'll find d missing cases n conditions easily.... [1]
13@asish...
both our answers are same... so v can be sure dat v r correct... [1]
341It is equivalent to
(x+1)(x+1/2) x (x-1/2)>0
Now its immediately obvious that the intervals are R-[(-1, -1/2) U (0, 1/2)]
1answer is (-∞,-1)Ï
(-1/2,0)Ï
(1/2, ∞)
given problem can be rewritten as
(2x-1)/[x(2x+1)(x+1)]
so critical points are -1, -1/2, 0, 1/2
therefore it will be +ve in the above given range.
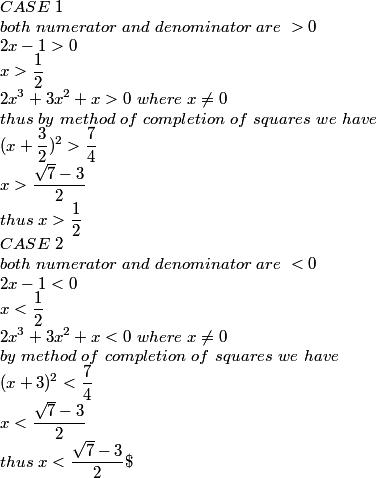
1@MAQ can you please tell me which cases i have missed because a fraction can be positive if both the numerator and the denominator are positive or negative
11@rahul1993
in case 1 in the denominator two inequalities can arise when u take x common outside cant it ??
1u have not missed any case but ur soving is wrong
2x3+3x2+x >0
x(2x2+3x+1) >0
x(2x+1)(x+1) >0
then from this case u get (-1, -1/2)Ï
(0, ∞)
so x >1/2
in second case
u get (-∞ ,-1)Ï
(-1/2, 0)
so u get (-∞ ,-1)Ï
(-1/2, 0)
so final answer will be union of both