functions TMH

adding more questions...
MULTI ANSWERS (ALL)
Q5. Let f(x) = \frac{\sqrt{sinx}}{1+\sqrt[3]{sinx}}. If D is the domain of f, then D contains
(a) (0,pi) (b) (-2pi,-pi) (c) (2pi,3pi) (d) (4pi, 6pi)

ans given: ac

Q6. Let f(x) = sin(\frac{\pi}{x}) and D₊ = {x|f(x) > 0}. Then D₊ contains

(a) (1/3, 1/2) (b) (1/5, 1/4) (c) (-1, -1/2) (d) (-pi -1/2)
ans given: abc

Q7. Let f(x) = x², g(x) = √x, tehn
(a) gof(-2) = 2
(b) gof(4) = 4
(c) gof(3) = 6
(d) gof(2) = 4

ans given: b

Q8. SOLVED (VERIFIED BY WOLFRAM ANS: 1/3)
\lim_{x\rightarrow 0}\frac{tanx-x}{x^2tanx}

ans shud be 1/3 naa?

ans for the 8th question must be 1/3 .. and in the sevenh question g(x) is not mentioned if we take the second function as g(x) then ul get gof=x so answer is a and b .. since f(x) is an even function f(2)=f(-2)=4 ...

Q5. R1 => sinx ≥ 0
This implies x → [0, pi]

R2 => 1 + ³√sinx ≠0
This implies sinx ≠³√-1
Assuming real domain, sinx ≠-1
So x ≠(2n + 1)pi/2, n = 1,2,3..

So [0, pi] and [2pi, 3pi] possible.

ans for the fifth one is a b and c ... sinx>0 is the only condition ..

In 5th option B is also possible...

is the answer for the third question (-52,-1]

7.gof=√(x²)=|x|
from the given options,a and b are thus correct.
dont know why only b has been given.

Q3.

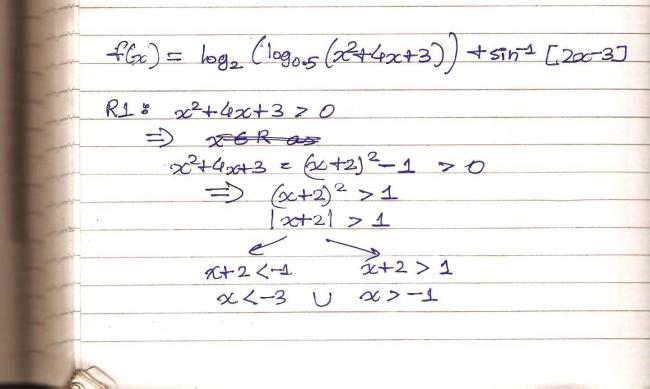
Final Domain : x → (-√5/2, -√2] U [√2, √5/2)

for q 2,the function
i)is not onto as it takes values in [1,∞)
ii)is not one one as any value a and -a have the same y(a²+1)
the function clearly is not invertible.