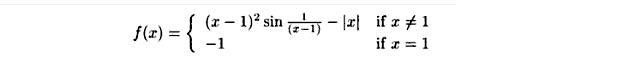LIMIT

5 Answers

fucontinuous at x=1

i feel yes it is!book says not!

f(-h) = -1
f(h) = 2h+1

where h is a "small" positive number (however small u want)

g(h)=f(h) + f(h) = 2h+1 + 2h+1 = 4h+2
g(-h) = f(h) + |f(-h)| = 2h+1 + |-1| = 2h+2

limiting value from both sides = 2

g(0) = f(0)+|f(0)|=1+1=2

u must have done some silly mistake!

LOL......i missed the definition of function n yeah very silly of me[3]

Nice Solution

√ √ || {} [] () → ~ ^ x x x → → ln log lg lim lim cos sin tan cot arcsin arccos arctan

∫ ∫ ∫ ∫ ∫ ∬ ∬ ∬ ∬ ∬ ∭ ∭ ∭ ∭ ∭ ⨌ ⨌ ⨌ ⨌ ⨌

∑ ∑ ∑ ∑ ∑ ∮ ∮ ∮ ∮ ∮ ∏ ∏ ∏ ∏ ∏ ∐ ∐ ∐ ∐ ∐

+ - × ÷ ± ∓ = ∪ ∩ ≠ ∼ ≈ ∅ ∀ ∃ ∈ ∋ ∝ ≤ ≥ ≃ ≅ ≡ < > ≪ ≫ ⊂ ∉ ⊃ ⊆ ⊇ ⇒ ⇐ ⇔ ⇒ ⇐ ⇔ ⇑ ⇓ ⇕ → ← ↔ → ← ↔ ↑ ↓ ↕ ↖ ↗ ↘ ↙ ↪ ↩ ⇀ ↼ ⇁ ↽

° ∂ ∞ α β γ δ θ λ μ ν ξ π ρ σ φ ω ε ƒ κ τ υ Γ Δ Λ Σ Π Ω