1that is nCr
1) evaluate \sum_{r=1}^{667}\binom{2000}{3r-1}
2)evaluate \sum_{r=1}^{2008}r.r!
3)find \prod_{r=1}^{45}(1+\textup{tan} (\textup{r}))
-
UP 0 DOWN 0 0 12
12 Answers
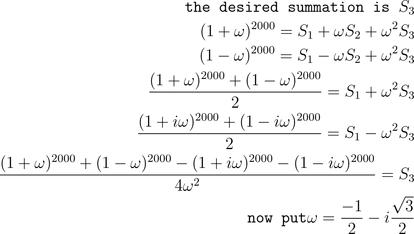
39q2 )
add and subtract r!
r.r! + r! - r! = (r+1)!-r!
and Σ [(r+1)!-r!] where r runs from 1 to 2008
= 2009!-1!
39i just used normal multiplication seeing the pattern in the question
11For the 1st sum, here is the method,
Since the difference between the suffices of the terms is 3, we look for the cube roots of unity.
In general,in such sums if the difference is n, then look for the nth roots of unity.
We have the following....
2^n=\sum_{r=0}{\binom {n} {r}...........(1)
(1+\omega)^n=\sum_{r=0}^{n}{\binom{n}{r}\omega^r}.........(2)
(1+\omega^2)^n=\sum_{r=0}^{n}{\binom{n}{r}\omega^{2r}}.........(3)
Multiply (1) by 1 , (2) by omega and (3) by omega^2.
2^n+\omega (1+\omega)^n+\omega^2(1+\omega^2)^n=\sum_{r=0}^{n}{\binom{n}{r}\left\{1+\omega^{r+1}+\omega^{2r+2} \right\}}
Plug in r=3k-2, to have rhs of the form
3\left(\binom{n}{2}+\binom{n}{5}+........ \right)
L.H.S becomes 2^n+\omega(1+\omega)^n+\omega^{n+2} =2^n+\omega^{2n+1}+\omega^{n+2}
Put n=2000, it's done.