modulus function

(1) find value of x in | | | |x| - 2| - 2|- 2| = | | | |x| - 3| - 3|- 3|
where | x | denote Modulus function.

(2) find real solution of the equation [x]*{x} = x.
{ } = fractional part function.

[ ] = Integer part function.

#Mathematics #Algebra

2 Answers

2 )

a graphical approach

graph of y =[x]*{x}

and graph of y=x

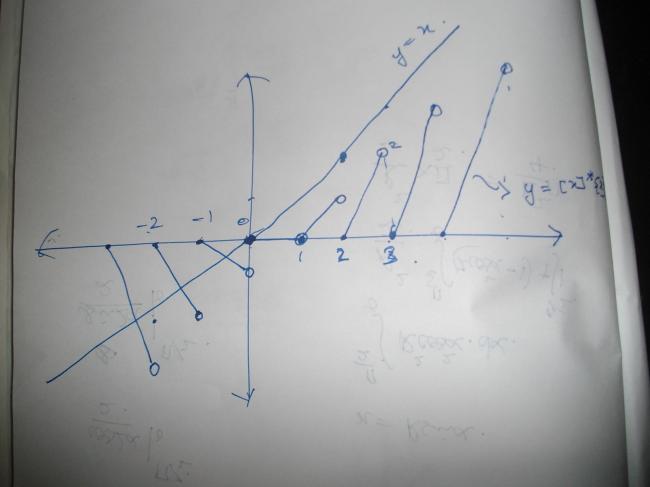
we can see infinite number of solution

2 > Given ,

x [ x ] - [ x ] ² = x ...............using the fact { x } = x - [ x ]

or , [ x ] ² = x [ x ] - x = x ( [ x ] - 1 ) = x . ( an integer ) .................. ( 1 )

Clearly , [ x ] ² is an integer .

So , R . H . S of the said relation ( 1 ) must also be an integer , which must be a perfect square .

For that , x must be an integer , or x must be a proper fraction whose denominator must divide [ x ] - 1.

But if x is an integer , then [ x ] = x .

So , the given relation yields x = 0 as the only solution in integers .

But the other case yields infinite solutions .

√ √ || {} [] () → ~ ^ x x x → → ln log lg lim lim cos sin tan cot arcsin arccos arctan

∫ ∫ ∫ ∫ ∫ ∬ ∬ ∬ ∬ ∬ ∭ ∭ ∭ ∭ ∭ ⨌ ⨌ ⨌ ⨌ ⨌

∑ ∑ ∑ ∑ ∑ ∮ ∮ ∮ ∮ ∮ ∏ ∏ ∏ ∏ ∏ ∐ ∐ ∐ ∐ ∐

+ - × ÷ ± ∓ = ∪ ∩ ≠ ∼ ≈ ∅ ∀ ∃ ∈ ∋ ∝ ≤ ≥ ≃ ≅ ≡ < > ≪ ≫ ⊂ ∉ ⊃ ⊆ ⊇ ⇒ ⇐ ⇔ ⇒ ⇐ ⇔ ⇑ ⇓ ⇕ → ← ↔ → ← ↔ ↑ ↓ ↕ ↖ ↗ ↘ ↙ ↪ ↩ ⇀ ↼ ⇁ ↽

° ∂ ∞ α β γ δ θ λ μ ν ξ π ρ σ φ ω ε ƒ κ τ υ Γ Δ Λ Σ Π Ω