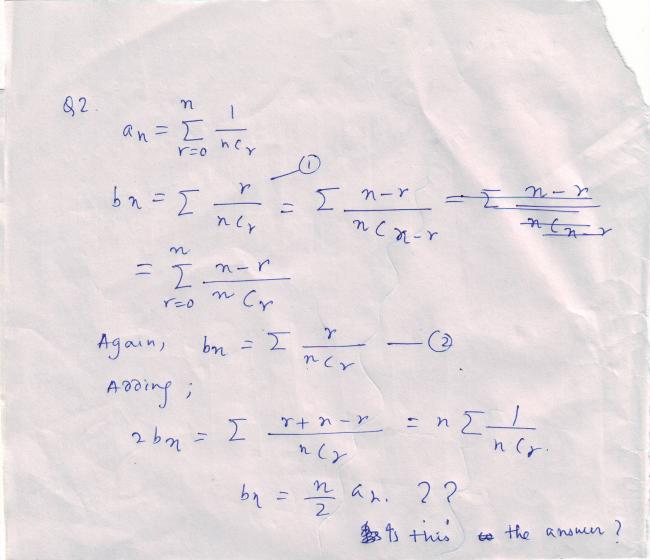P&C Basic Doubts

Q1. Every one of the 10 available lamps can be switched on to illuminate a certain hall. The total number of ways in which the hall can be illuminated is -:
a) 55
b) 1023
c) 2¹⁰
d) 10!

My Working : Each lamp can or cannot illuminate a hall, i.e., it has two states of working. So for n lamps, the hall can be illuminated in 2¹⁰ ways. But the answer is 2¹⁰ - 1....

Q2. If a_{n} = \sum_{r = 0}^{n}{\frac{1}{^{n}C_{r}}} then \sum_{r = 0}^{n}{\frac{r}{^{n}C_{r}}} equals?

Couldn't get this at all...

Q3. In a football championship, 153 matches were played. Every team played one match with each other. The number of teams participating in the championship is?
a) 17
b) 18
c) 9
d) none of these

Q4. The sides AB, BC, CA of a triangle ABC have 3, 4, 5 interior points respectively on them(interior and on them??). The total number of triangles which can be constructed using these points as vertices is :
a) 220
b) 204
c) 205
d) 195

Q5. All letters of the word EAMCET are arranged in all possible ways. The number of such arrangements in which no two vowels are adjacent to each other is :
a) 360
b) 144
c)72
d) 54

My Working : Total possible arrangements = 6!/2! as E repeats twice. Now vowels we have = E(2), A(1). So in 6 places, possible ways to arrange two vowels together = 5 x 2 = 10(EE and EA). So total ways = 6!/2! - 10 = 350 which is not the ans...