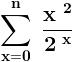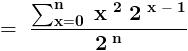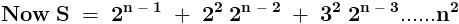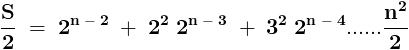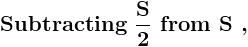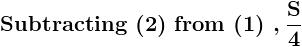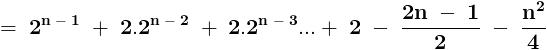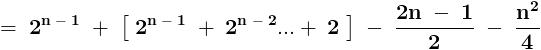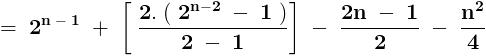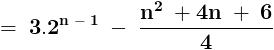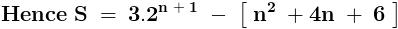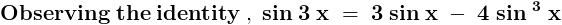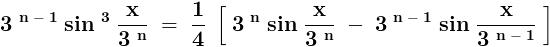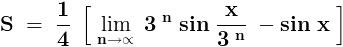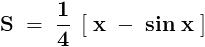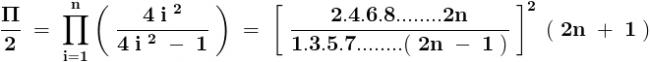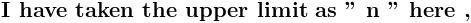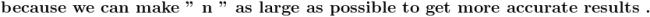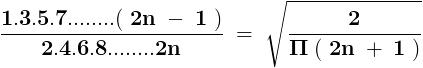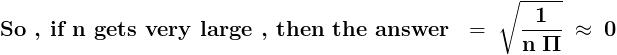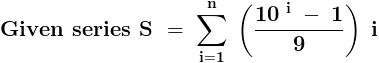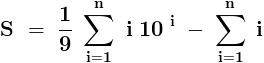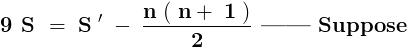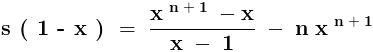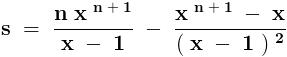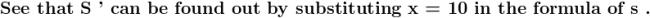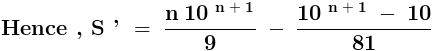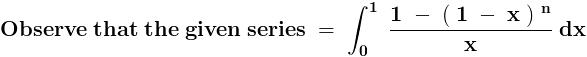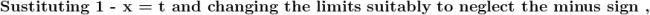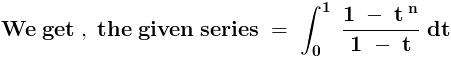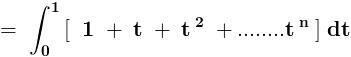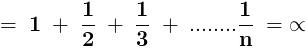1Q .11 > Is it needed that we use the fact given ?
long time since posted ques.............here are some for 2011 aspirants
daily updates.....
Day1
Q1 Express1*3*5*...*(2n-1)2*4*6*8....*(2n) as a2w where a is odd and w<2n
Q2 Prove that there is no prime number in sequence
10001,100010001,1000100010001,.....
Q3 Sum
^nC_1-\frac{1}{2}\ ^nC_2+\frac{1}{3} \ ^nC_3-....+(-1)^{n+1} \ \frac{1}{n} \ ^nC_n
Q4 Sum of the series
1+22+333+.....+n(11....1)
n
Q5\sum_{n=1}^{\infty}{\sum_{m=1}^{\infty}{\frac{1}{m^2n+n^2m+2mn}}}
Q6 Let f is a differentible function at x=a and f(a)≠0.
then calculate \lim_{n\rightarrow \infty}[\frac{f(a+1/n}{f(a)}]^n
Q7 \lim_{n\rightarrow \infty}\frac{1}{n^4}\prod_{i=1}^{2n}{(n^2+i^2)}^{1/n}
------------------------------------------------------------------------------------
Day2
Q8For k=1,2,3...caclulate
sin(\frac{\pi}{2k}).sin(\frac{3\pi}{2k}).sin(\frac{5\pi}{2k}).....sin(2[\frac{k+1}{2}]-1)\frac{\pi}{2k}
Q9 \sum_{n=1}^{\infty}{3^{n-1} \sin^3(\frac{x}{3^n})}
Q10 Prove that n^5/5+n^4/2+n^3/3-n/30 is an integer for n=1,2,3..
Q11 Given\int_{0}^{\infty}{\frac{sinx}{x}}dx=\pi/2
calculate\int_{0}^{\infty}{\frac{sin^2x}{x^2}}dx
Q12 \sum_{k=1}^{n}{\frac{k^2}{2^k}}
Q13 Find the largest integer n for which n3 + 100 is divisible by n+10?
----------------------------------------------------------------------------------
-
UP 0 DOWN 0 2 27
27 Answers
49ummm i cannot prove..
{cos\frac{x}{2^n}}=1-\frac{x^2}{n^2\pi ^2}
in another way...as we all know
\frac{sin x}{x}=1-\frac{x^2}{3!}+\frac{x^4}{5!}-\frac{x^6}{7!}....{maclaurin series}
but how does that help???
no way...[3][3]
1Q . 11 -
In all the integrations done below , the limits are from zero to infinity .
∫ sin 2 xx 2 dx
= - sin 2 xx + ∫ sin 2 xx dx . . . . . . . . ( After Part - Integrating )
Substituting " x = 2 z " yields ,
= - sin 2 xx + ∫ sin zz dz
= A + B . . . . . . . . ( Suppose )
Now put the limits.
Obviously , A = 0 .
And B is given to be Î 2 .
Hence the answer is Î 2 .
1DOUBT
evaluate
\int \frac{dx}{sin^n x + cos ^n x}
\texttt{CASE 1- when " n " is an even integer }
where n >2
\texttt{CASE 2- when " n " is an odd integer , where n > 1 }
34112) Easier way:
t_r = \frac{r^2}{2^r} = \left[\frac{r(r+2)}{2^{r-1}} - \frac{(r+1)(r+3)}{2^r} \right]+ \frac{3}{2^r}
Hence \sum_{r=1}^n t_r = \sum_{r=1}^n \left[\frac{r(r+2)}{2^{r-1}} - \frac{(r+1)(r+3)}{2^r} \right]+ \sum_{r=1}^n \frac{3}{2^r}
\left[3 - \frac{(n+1)(n+3)}{2^n} \right]+ 3 \left(1-\frac{1}{2^n} \right) =6 - \frac{n^2+4n+6}{2^n} as obtained above
34110) Easier way:
Let S = \frac{n^5}{5} + \frac{n^3}{3} + \frac{n^2}{2} - \frac{n}{30}
Then S-n = \frac{n^5}{5} + \frac{n^3}{3} + \frac{n^2}{2} - \frac{31n}{30}
= \left(\frac{n^5-n}{5}\right) + \left( \frac{n^3-n}{3} \right) + \left(\frac{n^2-n}{2} \right)
By Fermat's Little Theorem, each of the bracketed expressions is an integer.
Hence S-n is an integer which implies S is an integer
1Q 13 >
n 3 + 100
= n 3 + 1000 - 900
= { multiple of (n + 10 ) } - 900 ............ as x 3 + y 3 is always divisible by x + y .
Hence , to satisfy the given condition , 900 must be a multiple of n + 10 also .
We have to maximise n such that n + 10 divides 900 .
Clearly , n = 890
212)
S(k) = 1 + 10^4 + 10^8 + . . . + 10^(4k)
for some positive integer k.
Note the three following identities:
10^(4k+ 4) - 1 = (10^4 - 1)(1 + 10^4 + 10^8 + . . . + 10^(4k)),
10^(2k + 2) - 1 = (10^2 - 1)(1 + 10^2 + 10^4 + . . . + 10^(2k)),
10^(4k+4) - 1 = (10^(2k+2) - 1)( 10^(2k+2) + 1).
Combining these,
(10^4 - 1)(1 + 10^4 + 10^8 + . . . + 10^(4k)) = 10^(4k+4) - 1
= (10^(2k+2) - 1)( 10^(2k+2) + 1).
= (10^2 - 1)(1 + 10^2 + 10^4 + . . . + 10^(2k)) (10^(2k+2) + 1)
Since (10^4 - 1)/(10^2 -1) = 100 + 1 = 101, we have
(1 + 10^4 + 10^8 + . . . + 10^(4k))*101
= (1 + 10^2 + 10^4 + . . . + 10^(2k)) ( 10^(2k + 2) + 1)
Since 101 is a prime number, at least one of the two factors on the right hand side is divisible by 101. If k > 1, then for whichever of them is divisible by 101, the quotient will exceed 1; hence 1 + 10^4 + 10^8 + . . . + 10^(4k) is expressible as a product of two factors, each greater than 1. When k=1 we have 10001 as a factor which is composite (73*137).
1
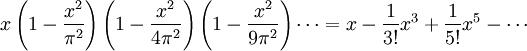.......... ( 1 )
which I have written after expressing " sin x " in Taylor series ( as stated by Organic ) ,
and equating the result with the expression of Infinite product for " sin x " .
Equate the co - efficients of x 3 in ( 1 ) .
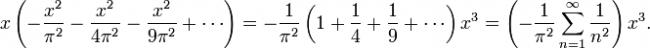.............. ( 2 )
or ,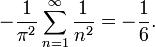 
From where we get ,
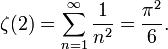
where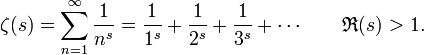 
is the Riemann Zeta Function .
P . S . --------- > I have 15 different mathods of finding ξ ( 2 ) .
1@organic
ur result combined with the above result helps in proving
\sum_{1}^{\infty}{\frac{1}{n^2}}=\frac{\pi^2}{6}
try
1poof for what kaymant sir has asked
writing sin xx in polynomial form
\frac{\sin x}{x}=\prod_{n=1}^{\infty}{(\frac{x}{n\pi}-1)(\frac{x}{n\pi}+1)}\\ \frac{\sin x}{x}=\prod_{n=1}^{\infty}{(\frac{x^2}{n^2\pi^2}-1)}
49sin\,x=2sin\,\frac{x}{2}.cos\,\frac{x}{2}=4sin\,\frac{x}{4}.cos\,\frac{x}{2}.cos\,\frac{x}{4}=8sin\,\frac{x}{8}.cos\,\frac{x}{2}.cos\,\frac{x}{4}.cos\,\frac{x}{8}
continuing for n times we get
sin\,x=2^nsin\,\frac{x}{2^n}.cos\,\frac{x}{2}.cos\,\frac{x}{4}.cos\,\frac{x}{8}.....cos\,\frac{x}{2^n}
let us multiply and divide this product by x and rewrite 2nx.sinx2n as sinx2nx2n
we get,
sin\,x=x\frac{sin\,\frac{x}{2^n}}{\frac{x}{2^n}}.cos\,\frac{x}{2}.cos\,\frac{x}{4}.cos\,\frac{x}{8}.....cos\,\frac{x}{2^n}
now if we let n→∞ by keeping x constant we will get.....
in formlimh→0sin hh=1
thus for infinite terms we get,
\frac{sin\,x}{x}=cos\,\frac{x}{2}.cos\,\frac{x}{4}.cos\,\frac{x}{8}.....\propto
\Rightarrow \frac{sin\,x}{x}=\prod_{1}^{\propto }{cos\frac{x}{2^n}}
1With all due regards , sir , I can prove it , but , it would take at least 30 minutes to latexify and post the proof . However , I shall prove it as you wish .
66@Ricky,
In #2, you say
"we can prove the following identity concerning infinite products:
\dfrac{\sin x}{x}=\prod_{n=1}^\infty\left(1-\dfrac{x^2}{n^2\pi^2}\right)
Prove it.
24alternate for Q6
we can write limit as
\lim_{t\rightarrow 0}[\frac{f(a+t)}{f(a)}]^{1/t}
For small t, f(a+t) and f(a) have same sign
=> log(\lim_{t\rightarrow 0}[\frac{f(a+t)}{f(a)}]^{1/t})=\lim_{t\rightarrow 0}(log[\frac{\left|f(a+t)\right|}{\left|f(a)\right|}]^{1/t})
=>\lim_{t\rightarrow 0}(\frac{log\left|f(a+t)\right|-log\left|f(a)\right|}{t})
This is ab initio defn of derivate of loglf(x)l at x=a
=> =>e^{\frac{f'(a)}{f(a)}}
**in a hurry used t in place of x...sorry for that [2]
24Sorry for error in Q1..corrected now
@gallarado,organic
Q3 ,6 are rite
49e^{f'(a)}.e^{\frac{1}{f(a)}}=e^{f'(a)+\frac{1}{f(a)}}
isn't it?
1
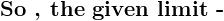
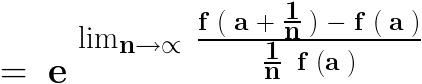
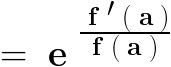
P . S . ------------------ Thanks to " ut10 " and " Organic " for pointing out a silly mistake .
