1)
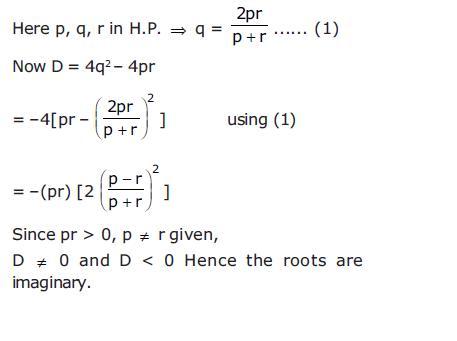
1. If p, q, r be in H.P. and p and r be different
having same sign, then the root of the
equation px2 + 2q + r = 0 will be
(A) Real (B) Equal
(C) Imaginary (D) None of these
2. If the equation (k – 2) x2- – (k –- 4) x- – 2 = 0
has difference of roots as 3 then the value
of k is
(A) 1, 3 (B) 3, 3/2
(C) 2, 3/2 (D) 3/2, 1
3.If the equation x2 + 2 (k + 1) x + 9k – 5 = 0
has only negative roots, then –find the values of k.
$\textbf{\mathbf{(1)} If $\mathbf{p,q,r}$ in H.P , Then $\mathbf{q=\frac{2pr}{p+r}}$ }$\\\\ $\textbf{Now discriminant $\mathbf{D= 4q^2-4pr =4\left(q^2-pr\right)}$}$\\\\ \mathbf{D=4.\left\{\left(\frac{2pr}{p+r}\right)^2-pr\right\}}$\\\\ $\mathbf{D=4.\frac{p.r}{(p+r)^2}.\left\{4pr-(p+r)^2\right\}}$\\\\ $\mathbf{D=-4.\frac{p.r}{(p+r)^2}.(p-r)^2}<0$\\\\ \textbf{bcz here $\mathbf{p}$ and $\mathbf{r}$ have same sign. and $\mathbf{p\neq r}$}\\\\. $\textbf{So Here discriminant is Negative, So Imaginary Roots.}$
Thanks Omkar for Nice solution.
(I have not seen your solution.)
\mathbf{(2)}:::$\textbf{If $\mathbf{\alpha}$ and $\mathbf{\beta}$ be the roots of Quadratic equation.$\mathbf{(k-2)x^2-(k-4)x-2=0}$}$\\\\ $\textbf{So $\mathbf{\alpha+\beta = \left(\frac{k-4}{k-2}\right)}$ and $\mathbf{\alpha.\beta =-\left(\frac{2}{k-2}\right)$}}$\\\\ $\textbf{Now Give that $\mathbf{|\alpha-\beta|=3\Leftrightarrow |\alpha-\beta|^2=9\Leftrightarrow (\alpha-\beta)^2=9$}}$\\\\ \mathbf{*\left(\alpha+\beta\right)^2-\left(\alpha-\beta\right)^2=4\alpha.\beta}$\\\\ $\mathbf{\left(\frac{k-4}{k-2}\right)^2-9=.\left(\frac{-8}{k-2}\right)}$\\\\ $\mathbf{2k^2-9k+9=0}$\\\\ $\boxed{\boxed{\mathbf{k=\frac{3}{2},3}}}$