Differentiability.

Q2. The function is not differentiable when cosec(x) = 2. Accordingly you'll find the general solution.
sin(x) = 1/2
=> sin(x) = sin(pi/6)
=> x = npi + (-1)ⁿpi/6

As the function is a composition of continuous functions which are continuous everywhere, so is the overall function.

i think it shud be

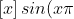LHD =\lim_{h\rightarrow 0}\frac{[k]sin(k\pi )-[k-h]sin(k\pi -h\pi )}{h}
=\lim_{h\rightarrow 0}\frac{ksin(k\pi )-(k-1)sin(k\pi -h\pi )}{h}

=\lim_{h\rightarrow 0}\frac{k(sin(k\pi )-sin(k\pi -h\pi ))+sin(k\pi -h\pi )}{h}

=k\pi cos(k\pi ) +\lim_{h\rightarrow 0}\frac{sin(k\pi -h\pi )}{h}

now cos(k pi ) = -1 for k =1 ,3,5,..
= 1 for k = 0,2,4 ..

thus cos(k pi ) = (-1)^k

also sin(k\pi -h\pi )= sin(h\pi)\; for \; k= 1 ,3,5,..
sin(k\pi -h\pi )= sin(-h\pi)\; for \; k= 0,2,4..

so sin(k\pi -h\pi )= sin((-1)^{k-1}h\pi)

so LHD=k\pi cos(k\pi ) +\lim_{h\rightarrow 0}\frac{sin(k\pi -h\pi )}{h}
=(-1)^{k}k\pi +\lim_{h\rightarrow 0}\frac{sin( (-1)^{k-1}h\pi )}{h}

=(-1)^{k}k\pi + (-1)^{k-1}\pi =(-1)^{k}k\pi - (-1)^{k}\pi

=(-1)^{k}k\pi + (-1)^{k-1}\pi =(-1)^{k}(k-1)\pi

Thanks for your valuable help.