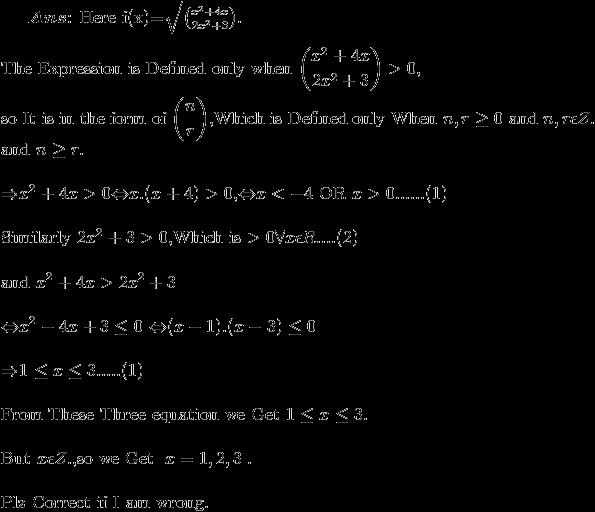Help me find the domain of the following functions.
1)f(x) = √(x2 - l x l - 2)
Here am getting 2 cases when x≥0 and x<0, for x≥0 am getting the value of x as x≥2
and for x<0 am getting x≥-2 and x≥1, please tell me how to write domain, given ans is x ε
R -(-2,2) and how this is the ans since the equality sign is greater than equal to 2 so won't
there be close brackets instead of open ones? Help me out to come out of this confusion,
please.
2)√(x12-x9-x4-x+1) why its answer is (-∞,∞),i.e, R ?
3)f(x)= log10log2log2/Ï€(tan-1x)-1. I this sum the
last step I got is (tan-1x)-1>2/Ï€, I don't know how to go ahead. [2]
4)f(x) =√(x2+4xC2x2+3)
5)f(x)=sin-1[2x2 - 3], [] denotes greatest integer function.
I face the problem while solving the problems that include greatest integer functions, I
don't know what to include and what not to, So please help me in this.
Tips and tricks are welcomed for soling the problems that include piecewise functions. [1]
391) f(x) = √x² - |x| - 2
We impose various restrictions(set theory ke mutaabik) as per definition of functions involved. Then we take intersection of those restrictions to get the domain.
R1 : Square root function. For √x, x ≥ 0.
So x² - |x| - 2 ≥ 0
=> |x|² - |x| - 2 ≥ 0 (As x² is the same as |x|²)
=> (|x| + 1)(|x| - 2) ≥ 0
Using the wavy curve method, we can easily see that |x| ≤ -1 U |x| ≥ 2 for the inequality to be satisfied(where U is union).
As |x| ≤ -1 is not possible, we discard it from the union(As any set U Null set = the set itself).
So |x| ≥ 2 or x ≤ -2 U x ≥ 2.
Hence R1 -> x ≤ -2 U x ≥ 2
R2 : Modulus function. For |x|, x ≥ 0.
Here simply x ≥ 0.
Now taking intersection, R1 ∩ R2 => x ≥ 2 is the domain of the function...
2) Dunno bout this one...it never came right for me when I tried it.
3) You have to be careful of the base of the logarithms here.
As per def of log function,
log2log2/π(Y) ≥ 0 where Y is (tan-1x)-1 for simplicity.
=> log2log2/π(Y) ≥ log21
=> log2/π(Y) ≥ 1
=> log2/π(Y) ≥ log2/π(2/π)
=> Y ≤ 2/π [Note the inequality change, this is because logarithm base was smaller than 1].
=> 1/tan-1x ≤ 2/π
I think we can cross multiply. This is because 1/tan-1x will have to be positive anyway, for the rest of the logs to work. Sooo...
=> tan-1x ≥ π/2...
Feeling sleepy...lol...I'll be back for the rest later [13]
11) case 1 .. x>0 then
f(x)=√x2-x-2
x2-x-2>= 0
(x-2) (x+1) >= 0
so, x E (-∞,-1] U [2,∞) but we have taken x>0 ,hence
x E [2.∞) ........1
case 2....x<0 then
f(x)=√x2+x-2
x2+x-2>= 0
(x+2).(x-1) >=0
x E (-∞,-2] U [1,∞) but we have taken x<0 .so
x E (-∞,-2] .........2
using 1 and 2
x E (-∞,-2] U [2,∞) .............3
or x E {R - (-2,2)}
OPEN BRACKETS BECAUSE -2 AND 2 ARE INCLUDED IN THE DOMAIN OF THE GIVEN FUNCTION AS U CAN SEE FROM EQ. 3
12)
either question or answer is wrong because putting x =1 we get i (iota)
15)
here cases arise as we get -1=< [2x2-3]>=1 in sin-1[2x2-3] ...so.....
0 =< 2x2-3 < 1..........eq.1 we get sin-10
-1 =< 2x2-3 <0...........eq.2 we get sin-1-1
2x2-3= 1...........eq.3 we get sin-11
2x2-3= -1..........eq.4 we get sin-1-1
2x2-3= 0..........eq.5 we get sin-10
now find out x using these equations.....
394) For this one, we'll have a bunch of tricky restrictions. x² + 4x and 2x² + 3 will have to be positive integers. I won't be going into non-integer factorials, because those require gamma functions/integrals...not sure whether those are in JEE syllabus.
R1 : Square root function.
x² + 4xC2x² + 3 ≥ 0
=> Always true. x belongs to the integer set Z+.
R2 : Positivity of numbers being operated on by C.
x(x + 4) > 0
=> x < -4 U x > 0
AND
2x² + 3 > 0
=> x can be any value.
Taking intersection, finally R2 is x < -4 U x > 0.
R1 ∩ R2 gives the domain as integers less than -4 and greater than 0. If there is a better way to solve this, I'd love to know..
5) For the GINT function, here's an exhaustive collection of properties -: http://www.targetiit.com/iit-jee-forum/posts/greatest-integer-function-exhaustive-collection-pl-13636.html. Check out the last(mine) post too.
f(x) = sin-1[2x² - 3]
R1 : Arcsin input always lies between -pi/2 to pi/2.
-pi/2 ≤ [2x² - 3] ≤ pi/2
We check these out separately.
[2x² - 3] ≥ -pi/2
The equal to part is not possible as the LHS is an integer and the RHS is not.
So [2x² - 3] > -pi/2 or > -1.57
This can be written as [2x² - 3] ≥ -1 so that both sides are integral.
For the output of the GINT function to be an integer greater than or equal to -1, 2x² - 3 ≥ 0 OR 2x² - 3 = -1.
So x ≤ -√3/2 U x ≥ √3/2 U {1, -1}
Similarly do [2x² - 3] ≥ pi/2 and take the intersection of both cases to get the domain...phew.
23for 2nd one may be u did some mistake while typing the sumas x = 1 is clearly not in domain
pritish for 5th one u made a mistake
-1 ≤[2x2 - 3 ] ≤1
so -1 ≤ 2x2 -3 < 2
1 ≤ x2 < 5/2
so x≡ (-√52 , -1] U [1 , √52)
233rd one
y= log_{10}log_{2}log_{2}log_{2/\pi}(\frac{1}{tan^{-1}x})
\Rightarrow log_{2}log_{2}log_{2/\pi}(\frac{1}{tan^{-1}x})>0
\Rightarrow log_{2}log_{2/\pi}(\frac{1}{tan^{-1}x})>1
\Rightarrow log_{2/\pi}(\frac{1}{tan^{-1}x})>2
\Rightarrow 0<(\frac{1}{tan^{-1}x})< (\frac{2}{\pi })^{2}
i.e \Rightarrow \infty>tan^{-1}x >(\frac{\pi}{2})^{2}
wich isnt possible ,
hence domain is an empty set ( if i didnt miss anything)
11Thanks a lot Pritish for the detailed explanation.[1]
@Mavarick, thanks a lot. You din make any mistake while solving the questions [1], nice explanation and solution[1]
You did a small mistake in the 5th question while solving
[2x2-3] < 2
here x will lie between -√(5/2) and √(5/2), Qwerty has given the solution too.
Thanks a lot Nihal and Qwerty [1]
11Yeah I made the mistake while typing 2nd one, Sorry.
It is as follows
√x12 - x9 + x4 - x + 1
11I got all the question now , yay yay except the 2nd one of course.
Thanks a lot lot friends [1][1]
23for 2nd one
case (i) | x | > 1
thus x8 + 1 + 1 > x8 + 1
thus x4(x8 + 1) + 1 > x (x8 + 1)
i.e x12 + x4 + 1 > x9 + x
hence all x satisfying |x| > 1 are in domain
now case (ii)
|x| ≤ 1
i.e x is a fraction
hence x4 > x9
1 > x
x12 > 0
hence
x12 + x4 + 1 > x + x9
hence all x satisfying |x| ≤1 also lie in the domain
hence all reals lie in the domain
