39\int{\frac{\sqrt{cos2x}}{sinx}}dx = \int{\frac{\sqrt{cos^2x - sin^2x}}{sinx}}dx = \int{\frac{\sqrt{1 - tan^2x}}{tanx}}dx
Let 1 - tan²x = t²
=> -2tanxsec²x = 2tdt
=> -tanxsec²xdx = tdt
Therefore,
\int{\frac{\sqrt{cos2x}}{sinx}}dx = -\int{\frac{t^2}{tan^2xsec^2x}}dt = -\int{\frac{t^2}{tan^2x(1 + tan^2x)}}dt = -\int{\frac{t^2}{(1 - t^2)(2 - t^2)}}dt
Now use fake substitution just for the purpose of partial fractions. Let t² = u.
\frac{u}{(1 - u)(2 - u)} = \frac{A}{1 - u} + \frac{B}{2 - u} \hspace{2mm} \textup{where A and B are found to be 1/2 and -2 respectively.}
So,
-\int{\frac{t^2}{(1 - t^2)(2 - t^2)}}dt = -[\frac{1}{2}\int{\frac{dt}{1 - t^2}} - 2\int{\frac{dt}{2 - t^2}} ]
Now it's easily integrable. Re-substitute t back when you get the answer.
1i guess the value of A wud be 1 instead of 1/2....thanx a lot anywayz
1
Aniket Ghosh Dastidar
·2010-05-17 00:21:56
1)∫ dx/{x2(x4+1)3/4}
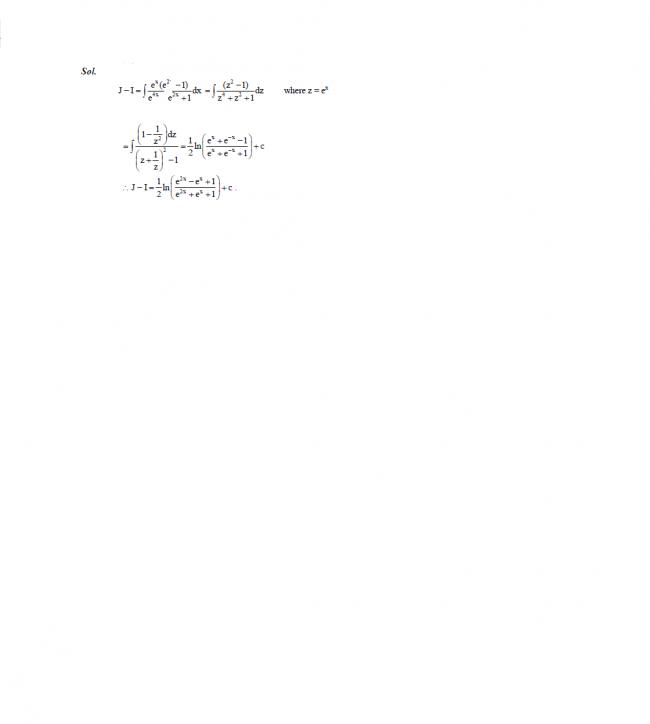
2) I=∫ex.dx/e4x+e2x+1 and J=∫ e-x.dx/e-4x+e-2x+1 then J-I=?
1\int \frac{dx}{x^2 ( x^4 + 1)^\frac{3}{4}}
\int \frac{dx}{x^2 .x^3( 1 + \frac{1}{x^4})^\frac{3}{4}}
\int \frac{dx}{x^5( 1 + \frac{1}{x^4})^\frac{3}{4}}
put
\frac{1}{x^4} = t
\frac{-4}{x^5} dx = dt
\frac{dx}{x^5} = \frac{-dt}{4}
integral reduces
\int \frac{ -dt }{4( 1+ t)^\frac{3}{4}}
which u can easily do !!!!
1
" ____________
·2010-05-17 00:43:48
2nd one
IIT JEE 2008 question
plz see dis
copied from fiitjee sols !!!!!!
1To post #4
Q1)
Put t4x4 = 1 + x4
→ t4 = 1 + x- 4
→ 4t3dt = - 4x- 5dx
→ t3 dt = - x- 5dx
now integral reduces to
\int x^{-2}(1+x^{4})^{-3/4}dx = \int x^{-2}(t^{4}x^{4})^{-3/4}dx
\Rightarrow \int x^{-5}t^{-3}dx=-\int t^{-3}(-x^{-5})dx=\int t^{-3}t^{3}dt=\int dt=t+c
where t = 4√1+x- 4
1
Manmay kumar Mohanty
·2010-05-17 00:51:15
Q2)
for I use ex = t and for J use e-x = u and then converting into perfect squares
use property \int \frac{dx}{x^{2}+a^{2}}=\frac{1}{a}tan^{-1}\frac{x}{a}+c
I hope that will do.
