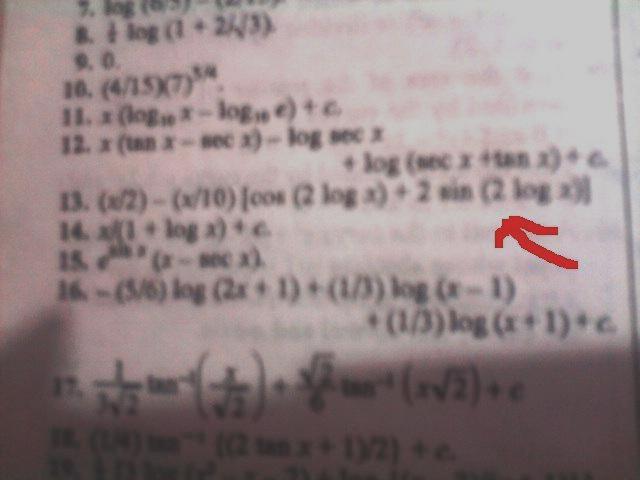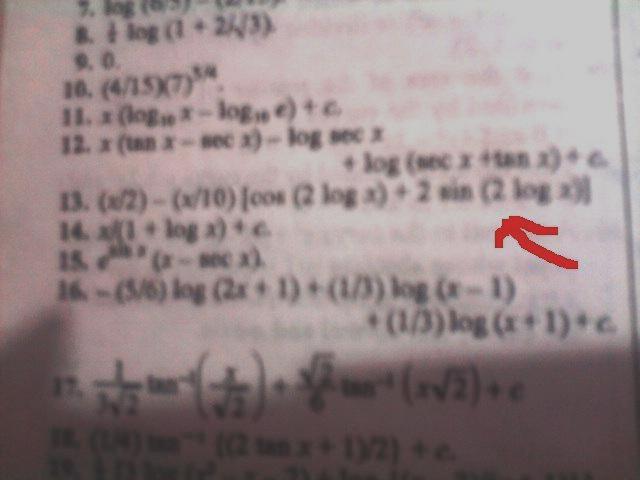1708\hspace{-16}\bf{\int \frac{\ln(x)}{(1+\ln(x))^2}dx}$\\\\\\ Let $\bf{\ln(x)=t\Leftrightarrow x=e^t\Leftrightarrow dx=e^tdt}$\\\\\\ $\bf{\int\frac{t}{(1+t)^2}.e^tdt=\int \frac{(t+1-1)}{(1+t)^2}.e^tdt}$\\\\\\ $\bf{\int \left\{\frac{1}{(1+t)}-\frac{1}{(1+t)^2}\right\}.e^tdt=\frac{e^t}{(1+t)}+C}$\\\\\\ Using $\bf{\int \left\{f(x)+\frac{d}{dx}(f(x))\right\}.e^xdx = e^x.f(x)+C}$\\\\\\ So $\bf{\int \frac{\ln(x)}{(1+\ln(x))^2}dx = \frac{x}{1+\ln(x)}+C}$
11) integrating by parts,(taking sin2(logx) as first function)
I=xsin2(logx)-∫sin(2logx) .....(1)
Now, ∫sin(2logx) = x.sin(2logx)-∫cos(2logx)
=x.sin(2logx)-∫1-2sin2(logx)
=x.sin(2logx)-x+2I
Now using (1)
I=xsin2(logx)-(x.sin(2logx)-x+2I)
I=\frac{1}{3}\left(xsin^{2} (logx)-xsin(2logx)+x\right)
1708\hspace{-16}\bf{\int \sin^2(\ln(x))dx}$\\\\\\ Let $\bf{\ln(x)=t\Leftrightarrow x=e^t\Leftrightarrow dx=e^tdt}$\\\\\\ $\bf{\int \sin^2(t).e^tdt}$\\\\\\ Now Let $\bf{\mathbb{I}=\cos^2(t).e^tdt....................(1)}$\\\\\\ and Let $\bf{\mathbb{J}=\sin^2(t).e^tdt....................(2)}$\\\\\\ $\bf{\mathbb{I}+\mathbb{J}=\int e^tdt=e^t+C_{1}}$\\\\\\ $\bf{\mathbb{I}-\mathbb{J}=\int \cos (2t).e^tdt=\frac{4}{5}\left(2.\cos(2t)-\sin (2t)\right)+C_{2}}$\\\\\\ So $\bf{\mathbb{J}=\frac{1}{2}.e^t-\frac{2}{5}\left(2.\cos(2t)-\sin (2t)\right)+C}$\\\\\\ So $\bf{\int \sin^2(\ln(x))dx=\frac{x}{2}-\frac{2}{5}\left(2.\cos(2.\ln(x))-\sin (2.\ln(x))\right)+C}$
I dont know Whether it is Right or Not (means Calculation)
Thanks
