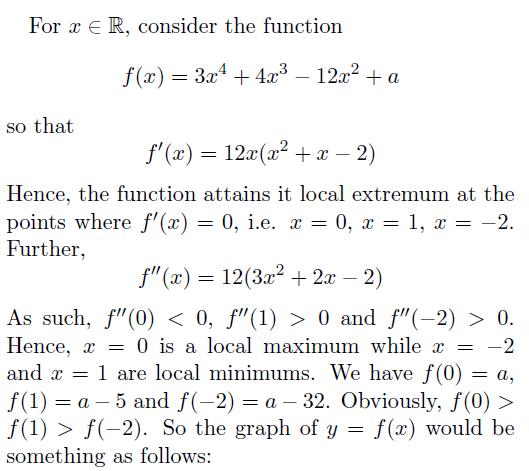
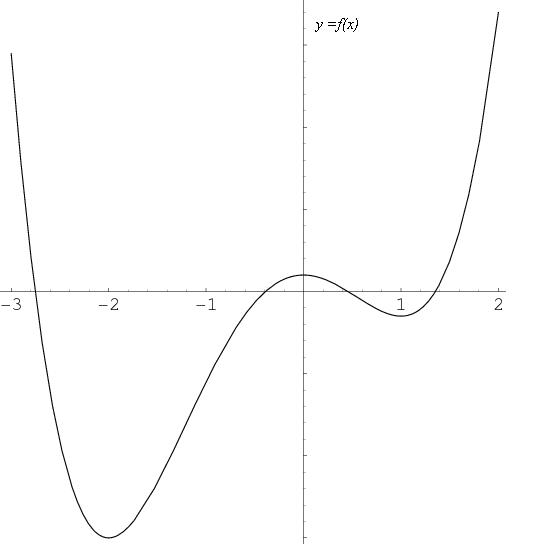
1) all values of a will have distinct roots thus a ≡ R
1) Find the values of a for which the eqn 3x4 + 4x3 -12x2 + a = 0 are real and distinct.
2) If f ' (sinx) < 0 and f '' (sinx) > 0 for x belonging to R, then find the intervals of monotonicity of the function g(x) = f(sinx) + f( cosx), where x belongs to {0, pie/2].
3) If f(1) = 0 and f'(x) > f(x) for all x ≥1 then prove that f(x) > 0 for x >1.
4) Find te min no. of rooots of the eqn xn - px+1 = 0 (p>0), where n belongs to N. Consider two cases n odd and n even.
-
UP 0 DOWN 0 0 7

7 Answers
Number 3 can be done by just applying the definition of the derivative from the limiting point of view....
f'(1)=f(1+Δh)-f(1)Δh>f(1), where Δh>0 but →0.....means f(1+Δh)>f(1)....this proves it....
Q3) I prove the generalized version: If f(x0) = 0 and f'(x) > f(x) for all x ≥ x0 then prove that f(x) > 0 for x >x0.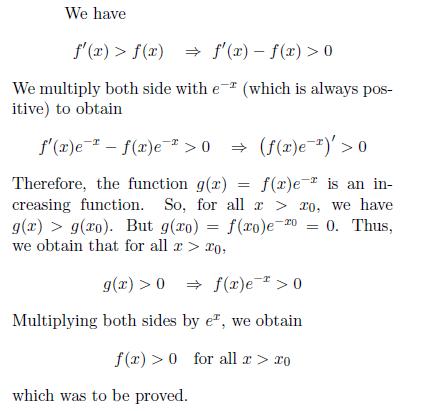
Thanx a lot kaymant sir
But plzzz solve the second one, plzzzzzzzz sir plzzz
g(x) = f(sin x) + f(cos x)
g'(x) = f'(sin x) cos x - f'(cos x) sin x
Consider the function h(x) = f'(sin x). Then h"(x) = f"(sin x) cos x>0 i.e. h(x) is an increasing function
1) x \in \left(0, \frac{\pi}{4} \right)
We have x < \frac{\pi}{2} - x
Then since f'(sin x) is increasing
f'(\sin \left(\frac {\pi}{2} - x \right) \right))> f'( \sin x) or
0> f'(\cos x)> f'( \sin x) \Rightarrow 0<-f'(\cos x) < - f'(\sin x)
Also in this interval 0<\sin x < \cos x
From the above two inequalities, we get
-f'(cos x) sinx <- f'(sin x) cos x or f'(sin x) cosx - f'(cos x) sinx <0
or g'(x) <0. That means g(x) is strictly decreasing in this interval
(ii) x \in \left(\frac{\pi}{4}, \frac{\pi}{2} \right)
Since g(x) = g \left(\frac{\pi}{2} - x \right) we have g'(x) = -g' \left(\frac{\pi}{2} - x \right) which tells us that in this interval g(x) >0 so that g(x) is strictly increasing
At x = π/4 of course g'(x) = 0.
4) Find te min no. of rooots of the eqn xn - px+1 = 0 (p>0), where n belongs to N. Consider two cases n odd and n even.
When n is odd, you will have one root minimum
When n is even, you will have 0 minimum number of roots
Think of the graph of x^n
and the graph of px-1
both should intersect for a root