11It is not that difficult
Ok here is the solution:
If we start solving the continued fraction from below we will find that-
1+1x=x+1x
1+1x+1x=1+xx+1=2x+1x+1
1+12x+1x+1=1+x+12x+1=3x+22x+1
After solving a few more of those we will find the other freactions are 5x+33x+2,8x+55x+3 and hence the
fibonnaci series is becoming visible as each of the fractions are of the form :
fn+1x + fnfnx + fn-1 where fn is the nth term of the fibonacci series
This is because:
1+1fn+1x + fnfnx + fn-1 =fn+2x + fn+1fn+1x + fn
thus we find that
fn+1x + fnfnx + fn-1 =x
on solving which we find the quadratic equation:
x2-x-1=0 hence x =1+/-√52
Another way of solving is if we assume that x+1x=x
The LHS does converge because:
fn+1x + fnfnx + fn-1 =2fnx + fn-1fnx + fn-1 =fnxfnx + fn-1 +1
Since fnxfnx + fn-1 converges the LHS converges
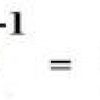1This simplifies to 11+x = x . Do u know the answer ? Is there no real solution to this question ??
30I guess its rather 1+\frac{1}{1+x}=x , which gives x^2=2 .
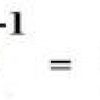1
sri 3
·2011-10-01 05:24:32
Sorry got confused with the question. I thought it was 11+11+ .... . And yeah #3 is correct.
341you know, before you do that neat trick, you have the responsibility to prove that that expression on LHS actually converges.
30
Ashish Kothari
·2011-10-01 05:33:33
Sir I never gave much thought seeing it under the IX-X section.
@ Prophet Sir - I have a very vague idea abt convergence. Can you please tell me where I can read about it, somewhat easy explanation?
11
Shaswata Roy
·2011-10-01 10:48:55
@Sri & @Ashish the question simplifies to 1+1x=x
This was the easy proof...can you give me another proof.(hint: start solving the continued fraction and you will find a form of a fibonnaci series)
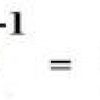1
sri 3
·2011-10-02 09:09:03
@ hsbhatt Sir : I dont know much (well almost anything) about that. Iam still in 11th. Even calculus is a bit much for me :P
@ Shaswata : Sorry. I need to know a lot more to give a proof using fibonacci series :\ :(
