area of 4 semicircles = \pi=area of square + area of shaded part
so area of shaded part = \pi -1
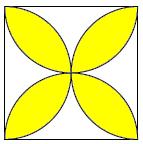
The side of the square is 1 unit and the circles have diameter as 1 unit..
-
UP 0 DOWN 0 0 6

6 Answers
firstly we numbering the regions , white region is called region A and yellow called B ..
SOLUTION
let the side of the suare is a then the radius become a/2 ..
remaining total area of REGION( A) = 2*(a*a - 2*pi/2*a*a/4) = x (let)
so for yellow area REGION (B) = a*a -x ,
final answer is =pi*a*a -a*a
Let the shaded portions be marked as 'a' and the unshaded portions as 'b'
Now, we have two equations
4a + 4b = 1 ------- (i)
2a + b = pi/2 ------ (ii)
on multiplying eqn. (ii) by 4 we have,
8a + 4b = 2pi ------ (iii)
On substracting eqn. (i) from (iii) we get,
so, 4a = 2pi - 1
or, a = (2pi - 1)/4
So, total area of shaded regions = 4a = 4 x (2pi - 1)/4 = 2pi - 1 Ans...!!