One topic troubling me in 3D coordinate geometry is the reflection of a line by a given plane ... can someone please help me .. nishant bhaiya please tell me the approach ??
1A sample sum asked in Target iit test few months back ....
A line passing through A(2,2,4) and parallel to line L ≡(x-2)=(y-1)=(z-2)/3 is incident of the
mirror plane x+2y+2z-5=0. The equation of the reflected ray is ..
The distance of the line L from the plane containing the incidence and the reflected rays is ..
1please try .... answer does not matter .. please give me the method to solve it !!
62ankit this is the thing where you have to use the direction cosines...
Here you know the unit vector perpendicular to the plane
also you know the unit vector along the line
now take a sum of v1+λv2 and take a unit vector v1+λv2
since it is a mirror image so
v1.v2 = (v1+λv2).v1
1bhaiya i tried but could not get it .. can u please solve the above given question so that i can get ur method !!
1anyone else who can show me the method is invited to do so ... :)
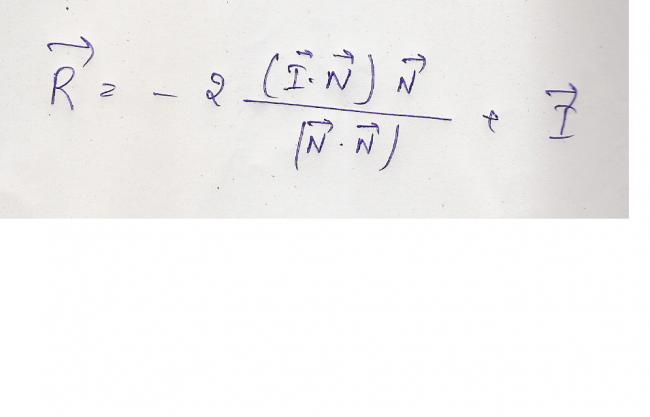
1well can give u a formula....derivation i 5 steps long...better to learn the formula....If u still want the derivation i can give u...
1kamal ... nishant bhaiya ..can u give me detailel co-ord approach .. please
62yes ankit the original method is very dirty and messy if i could say so...
You can even learn the forumula for this one...
62The method i said gives the set of unit vectors in the plane of the normal to the plane and the incident line
so then becasue these tow make the same angle (the new line and the old line) with the normal to the plane we take the dot product to be equall...
that is all :)
1.........KORBO...........LORBO.RE.......JITBO RE...............
