abhishek
yeh h , k kya liye hain???
find locus of center of circle which touches (y-1)2+x2=1 externally and also touches X axis.
-
UP 0 DOWN 0 1 13

13 Answers
(k-1)2+h2=(k+1)2
giving
x2=4y
also x=0
so 2 curves... are there....
(h,k) center of circle..
since it touches x axis so radius is k
distance from 0,1 =1+k (sum of radii)
abhishek i diasgree
(k-1)2+h2=(k+1)2
giving
x2=4y
also x=0
so 2 curves... are there....
IF (h,k) IS THE CENTER OF THE NEW CIRCLE THEN HOW COME THIS EQUATION FORMED????
diagram banao samaj me aa jayega
lhs is distance formula bw new circle centre n old one centre
rhs is k+1
k=radius of new n 1 radius of old
if (h,k )is the center then how would it satisfy the equation (y-1)2+x2=1
i show u the diagram
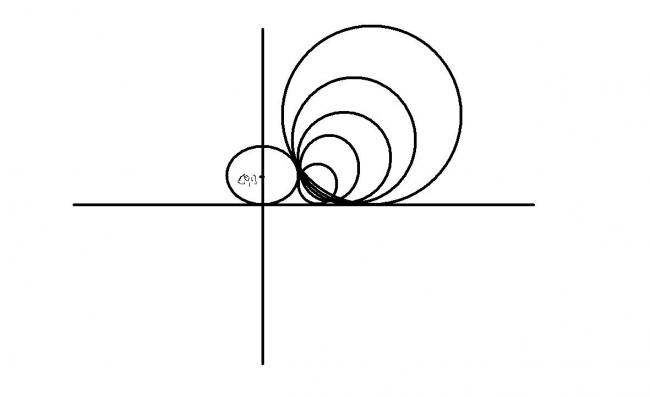
abhishek in #11u r saying that the center is fixed at (0,1) but the radius varies with y
please make me understand this thing
we r asked the centre of circle rite... so priyam assumed it to be (h,k).as the circle is touching x axis so its centre y coordinate tht s k =radius .now after that he used the 2nd cndition that s tangent to given circle having centre at (0,1) n radius 1 n thus distance bw centres is equal to 1+k(r1+r2)
r u cnvinced now/