Capacitance past IIT problem

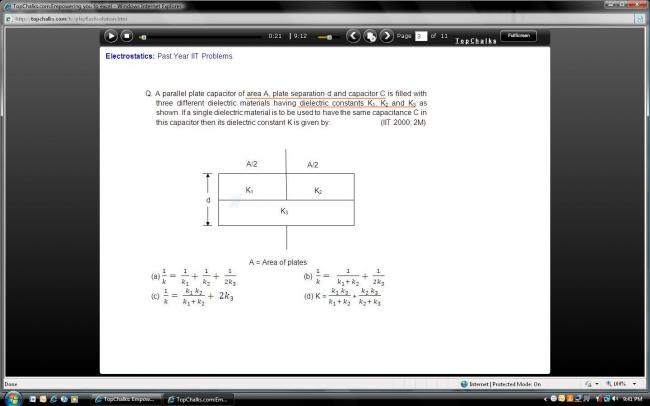
The solution given was faulty..anyone wanna try it?

C = \frac{\varepsilon _{o}S}{d}

where S = area

and C_{1}=C_{2}=\frac{\varepsilon _{o}\frac{S}{2}}{\frac{d}{2}}=C

and C_{3}=\frac{\varepsilon _{o}S}{\frac{d}{2}}=2C

now dilelctrics k₁ and k₂ are in parallel and k₃ is in series with the combination of k₁ and k₂

\Rightarrow k_{net}C_{net} = \frac{(k_{1}C_{1}+k_{2}C_{2})(k_{3}C_{3}) }{k_{1}C_{1}+k_{2}C_{2}+(k_{3}C_{3} }

\Rightarrow k_{net}C = \frac{(k_{1}C+k_{2}C)(k_{3}(2C)) }{k_{1}C+k_{2}C+k_{3}(2C)}

\Rightarrow k_{net} = \frac{(k_{1}+k_{2})(2k_{3}) }{k_{1}+k_{2}+k_{3}}

\Rightarrow \frac{1}{k_{net}} = \frac{k_{1}+k_{2}+2k_{3}} {(k_{1}+k_{2})(2k_{3}) }

\Rightarrow \frac{1}{k_{net}} = \frac{1}{k_{1}+k_{2}}+\frac{1}{2k_{3}}

gotcha
thankyou!