Q.1 In the figure shown , the middle plate is given a charge q. Each plate has an area A and separation b/w adjacent plates is d each.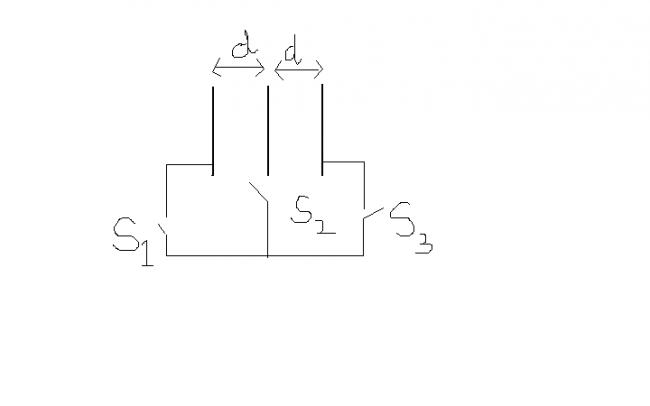
a) with all the switches open , the energy of the system stored in the region b/w the plates is ( q2d / 4pi (epsilon) A )
b) The p.d. b/w the left and right most plate is zero with all switches open
c) with S1 and S3 closed , S2 open , the energy of the system is ( q2d / 4pi (epsilon) A )
d) with S1 and S3 closed and S2 open , the energy of the system is ( q2d / 2pi (epsilon) A )
Q.2 In the given arrangement , the capacitors are initially uncharged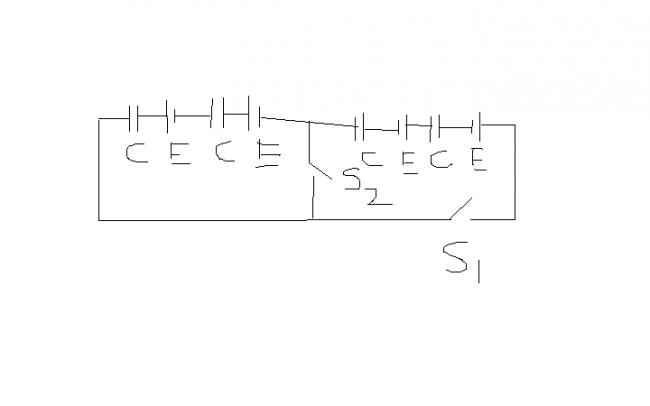
then find out :
a) The energy of leftmost capacitor (in steady state ) when S1 is closed and S2 is kept open.
b) The heat generated b/w t=0 and t=∞ , if S1 is closed and S2 is kept open
c) Long time after S1 is closed , S2 is closed. the heat generated in the circuit ( before it attains steady state ) is ?
-
UP 0 DOWN 0 0 1

1 Answers
V will be E.d=\frac{q/2}{\epsilon_0A}.d
*Because each capacitor on the left and right will have charge of q/2
Energy stored in each capacitor will be 1/2CV^2=1/2\frac{\epsilon_0A}{d}\times\left[ \frac{qd}{2\epsilon_0A}\right]^2 =1/2\times\left[ \frac{q^2d}{4\epsilon_0A}\right]
Since there are 2 capacitors, energy is \left[ \frac{q^2d}{4\epsilon_0A}\right]
The Pi given in the answer is wrong.. So if that is your confusion for the rest of the parts, then it is fine...