1by work energy theoram,
work done by all forces= change in KE of the body...
here, we can measure the potential difference between 2 pts if we move the particle quasistatically, from the first point to the other...
so,
Work done by us(external reagent) + Work done by the E field=∂KE
but here as the process is quasistatic, we mean ∂KE→0
or we have
work done by us=-(work done by E field)
by the definition of a conservative force, W=-∂U
or Work done by us=∂U
Work done by us=∂U= ∫(-E)dx {from infinity to x} as we apply an equivalent and opposite directional force to the E force, so as to keep the process of motion quasistatic,
or
∂U=∫-c/x3dx {infinity to x}
=c/2x2
cheers!!
66The fundamental idea one need to keep in mind is the fact the line integral for an electrostatic field over a closed path must be zero: i.e. for a electrostatic field \vec{E}, we have
\oint \vec{E}\boldsymbol{\cdot}\mathrm{d}\vec{\ell}=0
where the circle on the integral signifies integration over a closed path.
This idea leads us to conclude that the electrostatic field in an empty cavity inside a conductor must be zero. For any field line would have to begin and end on the cavity wall, going from a plus charge to a minus charge (as in the figure below)
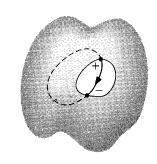
Letting that field line be part of a closed loop, the rest of which is entirely inside the conductor (where \vec{E}=0) the integral \oint\vec{E}\boldsymbol{\cdot}\mathrm{d}\vec{\ell} is obviously non-zero violating the above fact.
24Writing my soln again.in a bit more refined way......
consider any 2 points on cavity A and B
Now, B
VB-VA=-∫E.ds
A
where E is electric field existing in cavity &
path A→B is within the cavity
Since any 2 points on conductor are equipotential,
=>VB-VA=0
=> The path integral must be equal to zero irrespective of teh path followed b/w points A and B from within the cavity
Since path integral from A→B =0 ,
so Electric field within cavity is equal to zero at all points in cavity
9in #8 ur statement
Since any 2 points on conductor are equipotential, is right
but then when dealing inside a cavity its not always right
9
Celestine preetham
·2009-05-14 07:33:53
if we are getting same result why bother
both methods mite be right but go for shorter one
24
eureka123
·2009-05-13 11:31:30
@ cele...Q2 already solved in post#8.....check for errors...
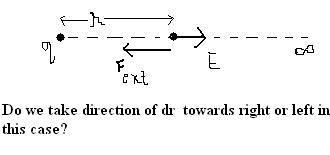
106so what is the meaning of dr vector. is it displacement vector. If so in this case shudnt we ACTUALLY write
dr' (disp. vector) = -dr
Hence E.dr' = E.dr'.cosÎ
= E(-dr)(-1)
= Edr
and then integrate
although u will get the same result
I mite be raking up a small issue. but fr me it is important. im sorry if those doubts are silly.
106
Asish Mahapatra
·2009-05-12 21:10:59
that means if we try to derive the potential due to a point charge q at a distance r:
then if we want to derive it from definition, i.e. potential = work done by external agent in a quasistatic process taking reference as ∞ and then integrating the work done as a unit positive charge is brought from infinity to r.
my doubt is this:
1i like one thing abt celes.....is that his cute pic...........
9
Celestine preetham
·2009-05-12 11:15:39
anyone trying Q2
its a nice standard concept to know and till now no one has posted xact explanation
1
gordo
·2009-05-12 11:14:50
yeah sorry for missing out dat 'q' cel....
1Assuming that field is only along the x-axis (because it is given only in terms of x)...
dV = -E.dx
dV = -\frac{c}{x^{3}}dx
\int_{V_{inf}}^{V_{P}}{}dV = -\int_{inf}^{x}{}\frac{c}{x^{3}}dx
V_{P} - V_{inf} = \frac{c}{2x^{2}}
Assuming V_{inf} = 0,
V_{P} = \frac{c}{2x^{2}}
9
Celestine preetham
·2009-05-12 11:04:25
@ ashish uve applied sign convention wrongly
dx means a small displacement along positive x direction
so E . dx = E.dx
9
Celestine preetham
·2009-05-12 11:02:14
gordo edits in ur post
replace dU by dU/dq ( WD per unit charge ie dV ) where dq is test charge
also V = c/2x2 + C
now V at ∞ = 0 (by definition) so C= 0
hence V = c/2x2
1Q1 V(x) = - ∫ E dx
Q2 ∫ E dA = Q/ε0 .. where Q is the charge contained in the cavity ...... since Q is 0 ... Electric field is 0 at all pts in the cavity
106
Asish Mahapatra
·2009-05-12 09:28:54
im saying that ∫U = -∫E.dr
So, if the particle goes in a direction opposite the electric field. Its pot energy should increase.
Here it is doing so.
So its pot energy shud increase
i.e. ΔU>0
hence ΔV>0.
So potential at that point must be posititve wrt infinity
however if u use wat ive ritten in #3 then u get potential at that point to be -ve.
9
Celestine preetham
·2009-05-09 02:27:45
i dont have hcv
i donno wat u r saying more clearly ?
106
Asish Mahapatra
·2009-05-09 02:22:50
if u have HCV please see wroked out problems 16 pg 118
106
Asish Mahapatra
·2009-05-09 02:22:08
cel: y pot has to be -ve? it is moving OPPOSITE to E isnt it? then pot shud increase i.e. is is positive wrt ∞.
9
Celestine preetham
·2009-05-09 02:19:36
ya pot has to be -ve compared to inf so charged body moves frm - ve to 0 ie x to inf which is correct
106
Asish Mahapatra
·2009-05-09 02:14:01
but celestine if #3 is right then we get potential negative. But if u analyse physically, the charged body moves in opposite direction of increasing electric field.So, the potential energy should increase hence potential at any point must be positive
9
Celestine preetham
·2009-05-08 22:55:27
sorry i was talking rubbish
field is cylindrically symmetric
24
eureka123
·2009-05-08 11:32:57
consider any 2 points in cavity A and B
Now,
B
VB-VA=-∫E.ds
A
where E is electric field existing in cavity
& path A→B is within the cavity
Since any 2 points on conductor are equipotential,
=>VB-VA=0
=> The path integral =0
=> Electric field within cavity is equal to zero at all points in cavity
106
Asish Mahapatra
·2009-05-08 11:06:04
but celestine : field is NOT spherically symmetric . It is directed only along +ve x-axis. So as we calculate potential by taking the particle from ∞ to that point x. displacement vector is opposite to electric field. hence angle is 180°. So why we write -∫Edx instead of-∫-Edx taking into account the dot product?
9
Celestine preetham
·2009-05-08 10:59:02
#3 field is spherically symmetric so dot product is same as product of magnitudes taking displacement along radial direction
24
eureka123
·2009-05-07 23:52:08
Q1 no asish..ankit is right
66
kaymant
·2009-05-07 23:33:01
@ankit, your argument is wrong. If Q=0, it simply proves that the flux through a closed surface is zero NOT THE FIELD. If you want to prove from your arguments that the field is zero, you need to take somehow that E outside of the integral.
