Electricity and magnetism - resistance and capacitance

1

lubu ·2010-04-08 22:10:39

Answer for d first one is \frac{R\left[(2K-1)+\sqrt{4K^{2}+1} \right]}{2K}

For d second one it is 1A, 2A, 3A for 1ohm, 4ohm, 5ohm resistor respectively.

UP 0 DOWN 0
Post on FacebookPost anonymously?

1

Manmay kumar Mohanty ·2010-04-08 22:28:30

Q2)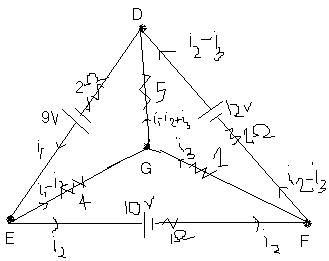

for mess EFGE
i₂ +10 + i₃ - ( i₁ - i₂) 4 = 0 â†’ i₁ - 5i₂ - i₃ = 10............(1)
for mess DEFD
2i₁ - 9 + (i₁ - i₂)4 + (i₁ - i₂ + i₃)5 = 0 â†’ 11i₁ - 9i₂ + 5i₃ = 9...............(2)
For mess FDGF
i₂ - i₃ -12 - (i₁ - i₂ + i₃)5 - i₃ = 0 â†’ 5i₁ - 6i₂ + 7i₃ = -12 ................(3)
for mess DEFD
2i₁ - 9 + 10 + i₂ + (i₂ - i₃) - 12 = 0 â†’ 2i₁ + 2i₂ - i₃ = 11 ..................(4)

R my equations correct ????

UP 0 DOWN 0
Post on FacebookPost anonymously?

1

Manmay kumar Mohanty ·2010-04-09 00:29:29

anyone please tell me if my equations r correct ?? Plese [2]

UP 0 DOWN 0
Post on FacebookPost anonymously?

1

kamalendu ghosh ·2010-04-09 01:57:16

in this figure we can always develop 3 matrices by loop analysis:

r11 r12 r13

r21 r22 r23
R=
r31 r32 r33

I= I1

I2

I3

E= E1

E2

E3

and put R*I=E

from here get I

r11=toral r in loop 1 ie r1+r2 ( from figure)

r12=-(r shared between loop 1 and loop 2 ) ie r12=-R2

sililarly r13=0

r22=R2+R3+R4 r21=-R2 r23=-R4

r33=R4+R5+R6 r31=0 r32=-R4

UP 0 DOWN 0
Post on FacebookPost anonymously?

1

kamalendu ghosh ·2010-04-09 01:59:12

if u r using my method stick to the current direction and emf convention as in fig

UP 0 DOWN 0
Post on FacebookPost anonymously?

11

SANDIPAN CHAKRABORTY ·2010-04-09 04:13:40

for 2 plz someone post the full solution....

i am getting these...

5 ohm = 1A

4 ohm = 0.7A

1 ohm = 1.7A

is their no other method than the matrix method (how 2 learn a new concept before 2 days of JEE) and kirchoff....plz plz help.. i am uploading my eqns... plz check whether they are correct or not....