ans. (4)
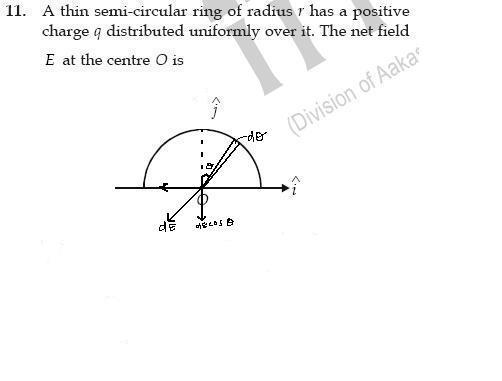
dE sinθ component of both halves cancel.
So E = ∫dEcosθ
where dE = kdq/r2
taking limits from 0 - Î /2,
we get E = q/2Π2ε0r2 along -ve y-dirn
ans. (4)

dE sinθ component of both halves cancel.
So E = ∫dEcosθ
where dE = kdq/r2
taking limits from 0 - Î /2,
we get E = q/2Π2ε0r2 along -ve y-dirn
hey one typing error... that should be
E = ∫ 2 dEcosθ if limits are taken from 0 to Π/2
or
E = ∫ dEcosθ if limits taken from -Π/2 to Π/2
however the answer given in the above post is correct.