Its 1/1+a + 1/1+b + 1/1+c ≥ 2 not = 2 I think we can still substitute this but the mistake is in 4 th step :(
I wiill put up some good questions of AM GM inequality only...
So that you all can try them.. some of themwill be very very easy.. others will be a bit more tough...
So try them all one by one..
I will try to post them in order of difficulty.
Easy one liners:
A1) \frac{a+b}{a}+\frac{b+c}{b}+\frac{c+a}{c}
A2) \frac{a-b}{a}+\frac{b-c}{b}+\frac{c-a}{c}
A3) Prove: a^2(1+b^2)+b^2(1+c^2)+c^2(1+a^2)>6abc
A4) Prove : AM HM inequality for 3 numbers a,b,c
-
UP 0 DOWN 0 1 68

68 Answers
"I 'm not too sure about my solution"
niether am I. u've messed up with the signs i think
we can't use substitutions like that
hmm, check your second step.
Its 1/1+a + 1/1+b + 1/1+c ≥ 2 not = 2
\frac{a+b+c+3}{3} \ge \frac{3}{ \frac{1}{a+1}+ \frac{1}{b+1}+ \frac{1}{c+1}} \ From\ AM-HM \\\\ Substituting \ in \ RHS \ denominator \ 2 \\\\ \frac{a+b+c+3}{3} \ge \frac{3}{2} \\\\ a+b+c \ge \frac{3}{2} \\ From \ AM-GM \ we \ have\ a+b+c \ge 3 \sqrt[3]{abc} \\ \frac{1}{2} \ge \sqrt[3]{abc} \\ \frac{1}{8} \ge abc I 'm not too sure about my solution. Wil try properly after english test tommorow :)
bhaiyya pls correct
C3
waise shayad (...)<=108 kuch
yes now sure....."max"(a.b^2.c^3) = 108
kya bhaiyya apne hi site ke test ka sawaal de diya woh bhi apni site ke hi studdents ko [3]
Skygirl.. the mistake in your method is that
AM GM holds when all the terms are equal..
your case equality never holds
because 3=a/b=b/c=c/a is not possible
2nd wala i am getting zeRO !
GIVEN STUFF = .[3 - (b/a + c/b + a/c)]
we minimise the (..) thing which is 3.
so ... given question is <= 0.
b/a + c/b + a/c > = 3
=> [3 + b/a + c/b + a/c] >= 6
[1]
shayad yeh behtar hai par us tarike se kyon nahi aaya is interesting point
1.) 1/4 .[3 + b/a + c/b + a/c] >= [3.b/a.c/b.a/c]1/4
=> [3 + b/a + c/b + a/c] >= 4.(3)1/4
=> given stuff >= 4.(3)1/4
hope i am corect ?
yeah sorry i forgt the six..
woh denominator mein hi kho jata hai har baar
baqi bhi sahi karte hain ab
f(x,y,z) = x/y + (y/z)1/2 + (z/x)1/3
let x/y=p, y/z= q2 , z/x=r3
now, g(p,q,r)= p + q + r
1/6 (p + q/2 + q/2 + r/3 + r/3 + r/3) ≥ [p.(q/2)2(r/3)3]1/6
=> (p+q+r) ≥ 6. [1/(27X4)]1/6 .... [pq2r3=1]
=> x/y + (y/z)1/2 + (z/x)1/3 ≥ 6. [1/(27X4)]1/6
=> f(x,y,z)min = 6. [1/(27X4)]1/6
right i hope....
well tapan i think there is a theorem of that kind..
I had read it long back dont remember now..
for symmetric functions, maxima minimas do exist when the terms are all equal.. and that is true from AM GM inequality alone.. (Because the equality holds when all terms are equal)
But I am not 100% sure... If i find that thoerem again i will let you know :)
A1 was much tougher than C1 probably [4]
joking [4]
but almost same not easy at all
A1 > = 6 after this A2 bcomes a sitter
A2 < = 0 (maybe i imagined the opposite sign but it is not a typo)
but please check these answers anyone
now i realize that A2 WAS THE BEST OF THE LOT
especially for lazy souls like me
KYA KARE PEOPLE DONT NOTICE UNTIL CAPITALS OR BOLD IS DONE otherwise i hate using caps or bolds
:O
im getting f(x,y,z) >= 6 \sqrt[6]{\frac{1}{27.4}}
(error pointed out by sky)
that is 27 x 4
definitely this cant be correct someone else try this please
I hav observed this (IT CAN B HORRIBLY WRONG) : "inequalities the equality occurs wen all the variables(if symmetrical) are equal"
IS THIS always TRU?? [7]
Solved Unsolved Details:
A1) Post 14 by Philip
A2) Post 15 by skygirl
A3) Post 21 by Rahul
A4) Post 23 by rkrish
C1) Post 11 by skygirl
C2)
C3) Post 22 by RKrish
App1)
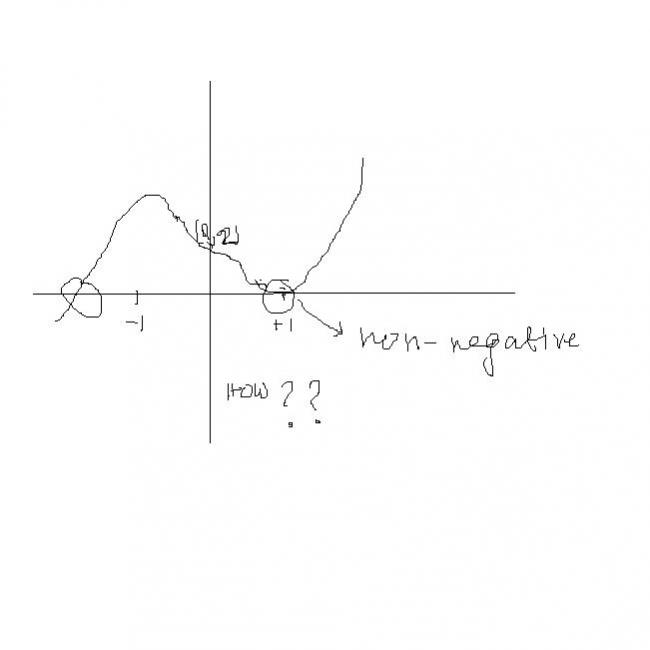
I'll add one: Prove that the polynomial P(x) = x3-3x+2 does not have any non-negative zeros
well.. from the above graph.... for a>=0, a3-3a+2>=0 always..
from am-gm ... thimking..
I dont know what came over me to write such baloney
Anyway what i meant was prove for non-negative a,
a3 ≥ 3a-2
@ sky [3][3][3]
@ prophet sir.... non negative zeros meaning non negative roots i believe
??
:'(
wat is non-negative 'zeros'...
non-negative part i understud... but wat is meant by zeros ?