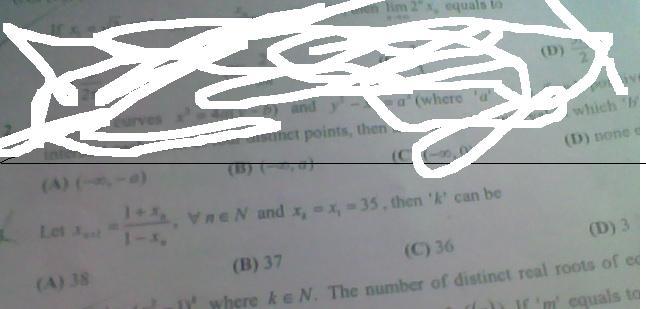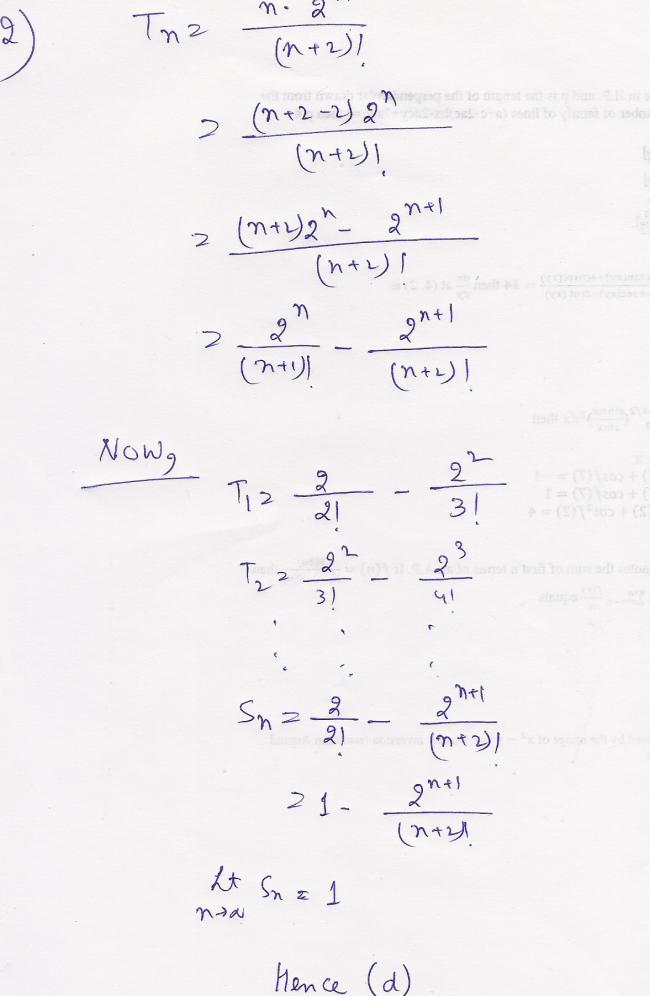bhaiya one of the unsolved is wat rkrish
2 has edited .. pls help wid dat
30 Answers
power play among all the options given see carefully only 37 is of the form 4n+1
x_{n+1}=\frac{1+x_n}{1-x_n}
x_{n+2}=\frac{1+x_{n+1}}{1-x_{n+1}}
x_{n+2}=\frac{1+\frac{1+x_{n}}{1-x_{n}}}{1-\frac{1+x_{n}}{1-x_{n}}}
x_{n+2}=\frac{2}{-x_{n}}
x_{n+4}=\frac{2}{-x_{n+2}}
x_{n+4}=\frac{2}{-\frac{2}{-x_{n}}}
x_{n+4}=x_{n}
thus k=4n+1 for all n
bhaiya one of the unsolved is wat rkrish
2 has edited .. pls help wid dat
playpower dude.. i have asked this more than once..
can you please post the questions that are unsolved with a better image or in text...
I guess i have real readability issues..
also if you could tell me exactly which questions to solve!
Is this the question ????
Prob 3
x_{n+1} = \frac{1+x^{n}}{1-x^{n}} for all n \epsilon N and x_{k}=x_{1}=35
Find k.
See at ur own risk !!
Put n=4
Total no. of ways = 4! = 24
a) 6(16-27+2) < 0 XXX
b) 12(9-8+1) = 24 √
c) 24(3-1) - 24(4-4) = 48 X
I solved it by some shortcut which I don't know is useful and correct always or not.
Playpower, in prob 1: does each urn contain n balls of each color or is total number of balls is n?
SOMEONE PLEASE HELP ABOUT PROBLEM NO. 1 .. THE SUM IS REWRITTEN ABOVE for the 2nd time .. FOR CLEAR VIEW
PROBLEM 1
n balls are picked one by one from 4 urns each containing n identical balls of green , red , white and blue colours . The number of ways , when no 2 consecutive pickings are from the same urn and balls of all the colours are picked , are
a> 6(4^{n-2}-3^{n-1}+ 2^{n-3})
b>12(3^{n-2}-2^{n-1}+ 1)
c>24(3^{n-3}-2^{n-4})-24(n-4)
d> none of these
I hope you got this :
N = 24 . 54 . ......
After this ,
you have to get
(p1+1)(p2+1)(p3+1)....(pn+1) = 105
105 = 3.5.7 = (2+1).(4+1).(6+1) √
= 15.7 = (14+1).(6+1) √
= 5.21 = (4+1).(20+1) √
= 3.35 = (2+1).(34+1) X (this is not possible as we know that N = 24 . 54 . ......... )
So, at the most 3 prime factors are possible (2,5 & a)
Hence, the cases mentioned above.
Prob 4
N = a1p1 . a2p2 . a3p3 . .... . anpn
(p1+1)(p2+1)(p3+1)....(pn+1) = 105 (which is odd)
So, p1,p2,p3, ... ,pn should all be even.
\Rightarrow N should be a perfect square.
N = 23 . 53 . ...........
So, definitely N is also divisible by 10000 (since powers of prime factors are even).
N = 24 . 54 . ...........
Now, 105 = 3 . 5 . 7
So, power of 2 & 5 cannot be 4 simultaneously.
So,
either N = 24 . 56 . a2
or N = 26 . 54 . a2
or N = 24 . 520
or N = 220 . 54
or N = 26 . 514
or N = 214 . 56
Therefore,
Total no. of odd factors can be = 12 , 8 , 20 , 4 , 14 or 6
Hence options A,B,C,D are correct.
no wat i am asking is, is there n balls of each color or n balls in each urn irrespective of the number of balls of each colour?