1for b replace ect in y(t) as u/(u-cx)
and replace t by (c)ln(u/(u-cx))
that will be the trajectory
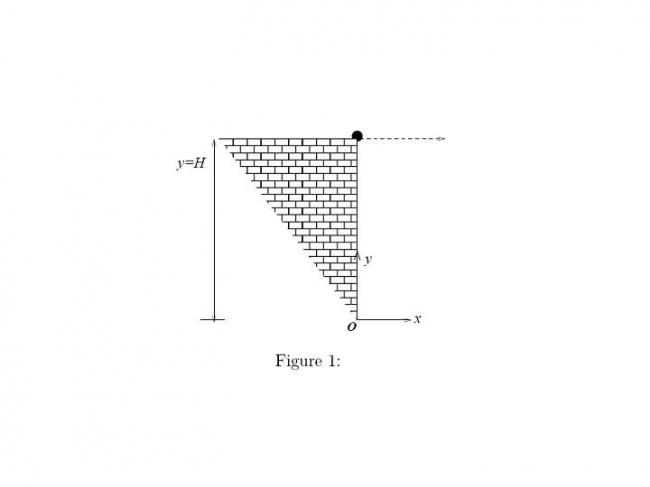Consider a ball which is projected horizontally with speed u from the edge of a cliff of
height H as shown in the Fig. (1). There is air resistance proportional to the velocity
in both x and y direction i.e. the motion in the x (y) direction has air resistance given
by the c vx (c vy) where c is the proportionality constant and vx(vy) is the velocity in the
x (y) directions. Take the downward direction to be negative. The acceleration due to
gravity is g. Take the origin of the system to be at the bottom of the cliff as shown in
Fig. (1).
(a) Obtain expression for x(t) and y(t).
(b) Obtain the expression for the equation of trajectory.
(c) Make a qualitative, comparative sketch of the trajectories with and without air
resistance.
(d) Given that height of cliff is 500 m and c = 0.05 sec−1. Obtain the approximate time
in which the ball reaches the ground. Take g = 10 m-sec−2
[ 4 + 3 + 2 + 3 = 12 ]
-
UP 0 DOWN 0 1 5
5 Answers
1
9yes
i think u ve got it
try the optics sum in olympiad question
that one is quite a toughie
3i think there shud be a mass term as welll!!!!!!!!!!!!!!!!! well in gettin a mass term!!!!!!!!!!!!!!
3rohans
takenaccn x,y as -cv and g-cv
-cv/m and g- cv/m!!!!!!!!!!!!!!!!!!!!!!!!!!
the rest of it is basic integrations!!!!!!!!!!!!:)