(a)my ans is √5rg
(b)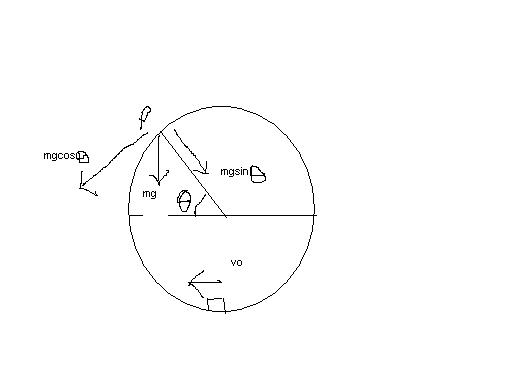
mgsinθ=mv2/r
rgsinθ=v2
1/2mvo=1/2mv2+mg(r+rsinθ)
sinθ=(vo22gr)/3gr
is this the ans??
the particle m in the fig is moving in a vertical circle of radius R inside a track.There is no friction.When mis at its lowest position,its speed is vo.(a)what minimum value vmof vofor wich m will go completely around the circle without losing contact with the track?(b)suppose vo is 0.775vm.the particle will move up the track to some pt at P at wich it will lose contact with the track & travel along the dotted path.Find angular position theta of point P.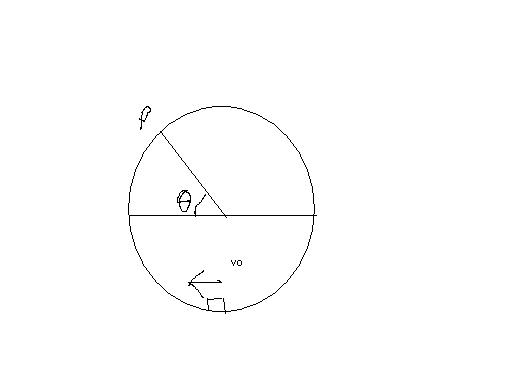
-
UP 0 DOWN 0 0 13

13 Answers
ans is written like this.
p is above horizontal by sin inverse 1/3...
is such an perfect result possible??
Answer for part (a) is √5gR.
Derivation is not there in HCV or other such books, but i have a book called JPH Physics for IIT JEE where it is written that minimum velocity to complete a full vertical circle is given by that expression. Derivation is not required, but that formula is an effective short cut for many sums.
Answer for part (b) is as below:
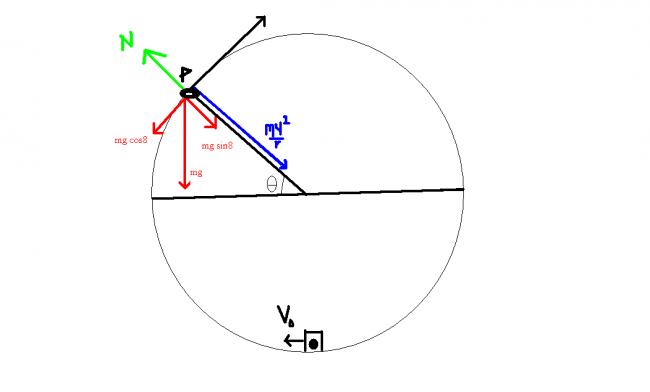
Here u hav to take normal reaction also. You ppl forgot normal reaction of the track.
Hence, mg sin8 - N = mv2r
So,
N = mv2r - mg cos8 --------------- 1
Also,
12 mv2 = mg (R - Rcos8) ---------------2 (Change in energy)
So,
mv2 = 2mgR(1-cos8)
mv2r = 2mg(1-cos8) ---------------------3
Substituting ean. 3 in eqn. 1,
N = 2mg(1-cos8) - mgcos8
For the body to slip, N = 0.
Hence,
2mg = 3 mgcos8
cos8 = 2/3
so the angle is cos-1 23
This means that horizontal distancehypotenuse (R) = 23
So, vertical distance should be √ [1 - (23)2 ]
so this means that sin8 = vertical distancehypotenuse (R) = √53
Hence the angle will be cos-1 (23) or sin-1 (√53)
There is some problem in the question i guess....
bhai ques mein toh likha hai"""what minimum value vmof vo""then vm shud contain vo alsoo.......i think
THIS QUESTION IS FROM RESNIC HALLIDAY OLD ONE CHP-energy conservation..check out if u can...
vm is a minimum value of vo it wont include vo and normal reaction at the top most pt and the pt at wich it loses contact is 0.for topmost pt it is 0 if we take limiting case.
kk mene question galat smajh liya thaa............................ √5rg will be d answer then.......
ya i got as for the first part bt second part is giving me bamboo...
can anybodygive a solution for the second part??
for second part
mgsinθ=mv2/R
(1/2)mv2+mgR(1+sinθ)=(1/2)mvo2=(5/2)gR.(0.775)2
actually, (0.775)2=0.6
that's why the answer is so simple
kooolio thnxx manish bhaiyaa................so the answer is sin inverse 0.3........