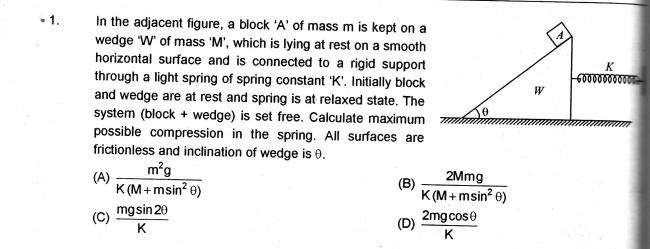
well for the first one if you got to choose any of the given options then it has to be (C) only.
check for θ = 0 , 90
7 Answers
1. For the first one, In exam I had got something like,
kX0 = Mmg sinθ cosθ(M+m sin2θ)
And Hence the maximum Compression should be Xmax = Mmg sin2θK (M+m sin2θ) .
So, I don't know which option and I ticked (b), which is wrong according to key given. I didn't even see the solution properly.
2. For the second one, We know that i = neAvdrift.
Now, as the electron moves, A decreases but since i is constant hence vdrift must increase.
Hence Statement 1 is correct.
Now assuming , no energy is dissipated in the motion of the electron, the increased KE must be provided by the PE of the electron - tube system. Hence, Statement 2 is correct.
So the answer may be B { I'm not sure of the explanation thing, Since first statement does explain second one but I'm not sure of the reverse case }
Q2.
Here we consider movement of an electron.
Assuming no. collisions and no loss of energy.
It is depicted that it is moving in a st. line(and not in a haphazard path) .it will only do so if it is under the influence of some +ve charge.
Hence its PE decreases.This is compensated by a inc. in KE.
Hence option a is correct.
@swaraj - there is no need of a +ve charge or electric field . the electron is simply moving with a vel.
My second explanation for the second question.
The question clearly says ' An ' electron is moving. Obviously, if it is a single electron, why should it's velocity or PE change at all.
Now, If we consider it to be corrected as stream of particles then I'll now go for ' C ' ie. 2nd statement is false. Ignore my previous explanation for this statement.
And yes I don't think my solution to first one is correct. I'm reworking on it now.