it is balanced on a nail
system shown of mass m is displaced in its plane by a small angle θ, find the period of oscillation  I wud essentuially want to know the approach to this type of questions? ANS : 2Ï€√2(√2)l/3g
I wud essentuially want to know the approach to this type of questions? ANS : 2Ï€√2(√2)l/3g
-
UP 0 DOWN 0 0 60

60 Answers
sinθ=θ for small angles
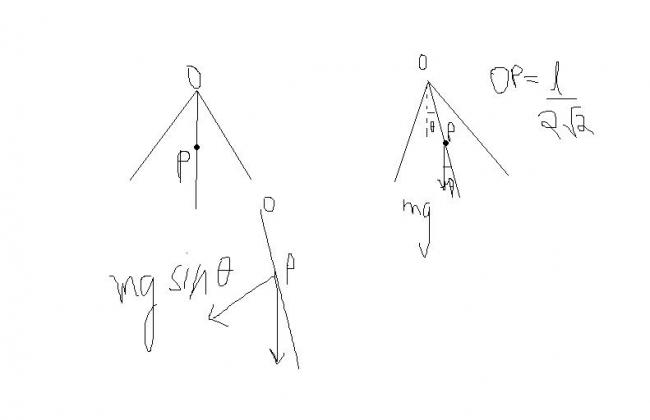
l/2√2 for distance between centres of mass and inertia
Tell me one thing avni is this thing handing from something or is it rotated about the dark point you have on the top?
Avni its tough since you will have to find the moment of Inertia of the object.
I donot know how to find th MOI of this object
How did you get that MOI pls explain there has to be a change in MOI for the ans to change?
Pls tell me the way you found the MOI
they are at perpendicular to each other. use perpendicular axis theorem
use torque reln
Iα = (mgl/2√2)θ (For small angles)
Substitute I = 1/3ML2
and u'll get ur answer
@vivek : "Iα = (mgl/√2)θ"
isme θ kyun aaya??
can u pl. xplain a lil i detail I need help in this topic pl. pl. pl. pl.
OH K!!!!
but hey can u pl. take it frm here on to gettin da time period, coz as i told I m bad at SHM
Avni..
Have you tried the energy method..?
Trust me it makes life much much much simpler most of the times...
Try doing that! :)
It takes away some understanding of physics though.. But it is good for exams.
Sir,
Can u pl. qoute the Energy method approach here!
Actually I m dying for an easy meth in SHM coz I hav struggling in this topic.
Ur help wud b invaluable, if I get a hand over this topic i can fancy my JEE chances.
Pl. Help
Onne doubt in vivek's meth,
sorry 2
1) while equatin torque on RHS how dus the factor of θ or sinθ cum??
2) α=ω2θ?? (pehle to kabhi nahi suna tha [2] )
For 2nd doubt.. first..
a=-ω2x hota hai naa... isi ka nakal hai for rotational motion...
Find α(bhai of 'acceleration in displacement') by simple rotation concept... if its proportionla of θ(bhai of 'x in displacement'..)
then it is SHM.. t=2pi/ω
COM is wrong in vivek...
its l/2√2
m/2 is mass of one rod..
moi=\frac{2}{3}\frac{m}{2}l^{2}
torque=mgsinθl/2√2
Iα=mgsinθl/2√2
\frac{2}{3}\frac{m}{2}l^{2}α=mgsin\theta \frac{l}{2\sqrt{2}}
now as θ is very very samll therfore sinθ=θ
\frac{2}{3}\frac{m}{2}l^{2}α=mg\theta \frac{l}{2\sqrt{2}}
ab thik hai... :)
that center line is imaginary..(rod like thing..to consider COM)
ok 1 sec...i will ask sky to do that.. nahi to khud kar denge.. :P