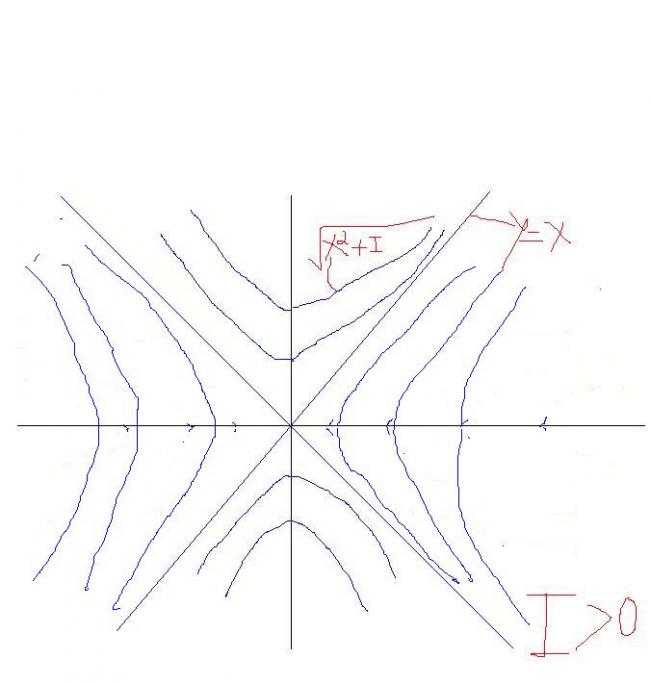
√x2-I are also tending to y=x..
a little error in graph.
20 Answers
yup subhash.. u are getting close :)
but there is more explanation needed :)
@subash :
at that time i was sleeping probably ;)
dont mind my "no-brainer"
i got it is a set of rectangular hyperbolas x2-y2=c
sorry for late reply.i have seen this time only
:P:P:P:P:P
lol...
seee that
x2=y2-1 satisfies the equation
so does
x2=y2-2
and
x2=y2-5
and
x2=y2+1
and
x2=y2+5
hope this is enuf to understand????
Bhaiyyah, I've completely lost track of what is happening [7][2]
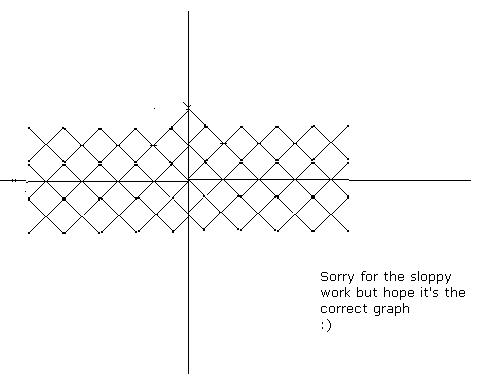
How did Abhi get that graph? And why is there no green background though it is correct?

(i know this is some miles away from the correct ans..
plz dun laf at my graphing skills... [2] [2] [2] [2])
and this one!
I know this is dirty.. and has a lot of things in it...
but this will only give u better thinking ability..
lol.. ok another hint...
think of the intervals for x as √N to √N+1
same way think of the y as between another two roots of perfect squares....
So you will easily get one point of y in each interval of y for each x between the given interval above....
this is not very simple... but then not as complex either....
There will be squares and rectangles of sides
√N-√N+1
and
√M-√M+1
Does this help????
alll points when x=y are solutions...
infact there are many more points..
what will they be?
(how i got the above graph...
)x2 = [0,1)
=> x = ± [0,1)
similarly for y...