21same here : 0^0 is not equal to 1 mere bhai..... it is nto defined so discont.
draw the graph of
y = {x}[x]
fractional power of x to the pwer of greatest integer of x!
-
UP 0 DOWN 0 0 18
18 Answers
21
106bhaiyya im taking a few examples:
1. x=-0.000001 {x}=0.9999999 [x]=-1 so y=1/0.9999999 ≈1
2. x=-0.999999 {x}=0.0000001 [x]=-1 so y=1/(0.0000001) ≈ infinity
3. x=-1.000001 {x}=0.9999999 [x]=-2 so y=1/(0.9999999)2≈1
4. x=-1.999999 {x}=0.0000001 [x]=-2 so y=1/(0.000001)2 ≈infinity
5. x=-2.000001 {x}=0.999999 [x]=-3 so y=1/(0.9999999)3≈1
So, graph shud be....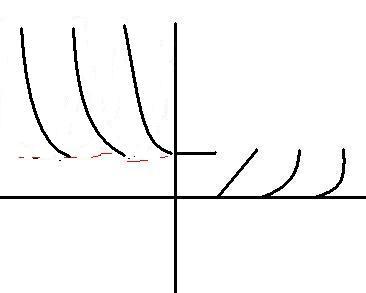
62you answer is correct between
(-2,-1), (-4,-3)
but I think it is not right in the intervals
(-1,0) and (-3,-1)
106bhaiyya i din get u..... [2] phir se dimaag chalaa gaya.[2]
62you repaired only the even intervals
what about the odd ones..
all the slopes should be downwards na?
62exactly my point asish.. you have drawn y is 1 very close to x=-1
check that :)
-0.99999999 is just near -1 but closer to the Y axis!
62Awesome try.. but I see a slight problem! A very slight one though!
the shapes will fall from infinity?
21hmmm..
maybe invert the pattern by abhi for x<0........
inverted patter as in style, the shape wud definitely change (i.e. it wudnt b mirror img of the graf portiion on da rite of y-axis...
I feel.... coz {-1.1} = 0.9 and [-1.1] = -2
so y1 = .9^(-2)
and for x = -1.2
y2=.8^-2
==> y2>y1
