1give d solution i need for dees question im confused
20 Answers
24before collison:
va=√2ga
vb=0
after collisoin
va=(m-2m/3)√2ga/(m+2m)
now calculate height
11See
F = kx for both
Acceleration for 1 will be kx/m1
Acceleration for 2 will be kx/m2
S1 = kx*t2/2m1
S2 = kx*t2/2m2
W1/W2= F * S1 / F * S2
W1/W2 = kx*t22m2/2m1kx*t2.
Therefore
W1/W2 = m2/m1
Is my answer rite?
Nishant Bhaiyya pls check my solution
1but how is W1/W2=m2/m1 plz xplain & even how is VA-VB=ERteta it isnt mentioned dat teta is small xplain
11ans for post #5 part 2
mgh = mv2/2
v is the velocity when it hit the ball
v=√2g*a
e = 1/3
e = v/v2-v1
v1 velocity of 2m v2 is velocity of m
mv = 2mv1 +mv2
v2 = v - 2v1
1/3 = v - v/3v1
v - 3v1= 3v
3v1 = 4v
v1= 2√2ga/3
2mgh = 1/2mv2
2g*h = 4*2*g*a/9*2
h =2a/9
1a straight uniform meal rod of length 3l is bent through right angle as shown.The bent rod is den placed on a rough horizontal table.a light string is attached to d vertex of d right angle. d string is den pulled horizontally so dat d rod translates at constant velocity den d angle alpha which d side 2l makes with string is:
3hi brinda ....
for ur first question ....
see watever body u pull after sum time the center of mass will be in line with the taut therad ............
do take the COMS od the individual rods and find position of COM ........
also use the above concept .......
then as nish byah says its all trigo.
1plz reply fast i need a complete solution if possible or else give at least hint which is useful
1
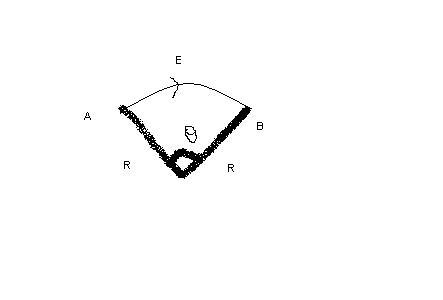fig shows an electric line of force which curves along a circular arc. d manitude of electric field intensity is same at all points on dis curve & is =E. if d potential at A is V, den d potential at B is:
12 blocks of masses m1 &m2 r connected by spring of constant K.The spring is initially compressed & d system is released from rest at t=0s d work done by spring on d blocks m1 & m2 be W1 & W2 respectively by time t. The speedes of both d blocks at time 't' r non-0 den d value of W1/W2 =?
1a stone is projected at an angle 45° with horizontal a bee follows d trajectory of d stone at a constant speed equal to d initial speed of stone.Neglect air resistance for motion of stone .den d magnitude of acceleration of bee at d topmost point of trajectory is?
a ball A of mass m is held at d top inside of a smooth circular tube of radius a/2 when it is released it collides with another ball B of mass 2m which is at rest at d bottom of d tube. if d coefficient of restitution is 1/3 , d height which A can attain after d collision is?
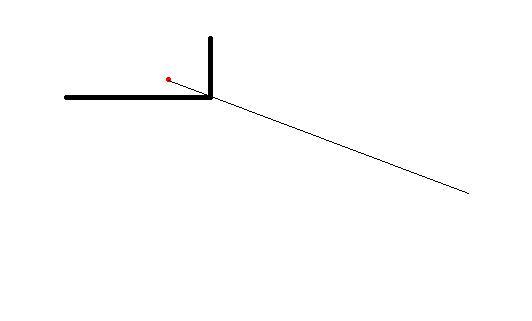
62see this diagram for more hint!
The red dot is the center of mass..
62good question!
this is by pure geometry
you have to locate the center of mass of the rod
is this hint sufficient?