3Only 6 views and still no answer :(
A horizontal plane with the coefficient of friction k supports two bodies : a bar and an electric motor with a battery on the block. A thread attached to the bar is wound on the shaft of the electric motor. The distance between the bar and the electric motor is equal to l. When the motor is switched on , the bar, whose mass is twice as great as that of the other body, starts moving with a constant acceleration w. How soon will the bodies collide.???
Ans:-- t=√2l/(3w+kg)
-
UP 0 DOWN 0 0 2
2 Answers
21
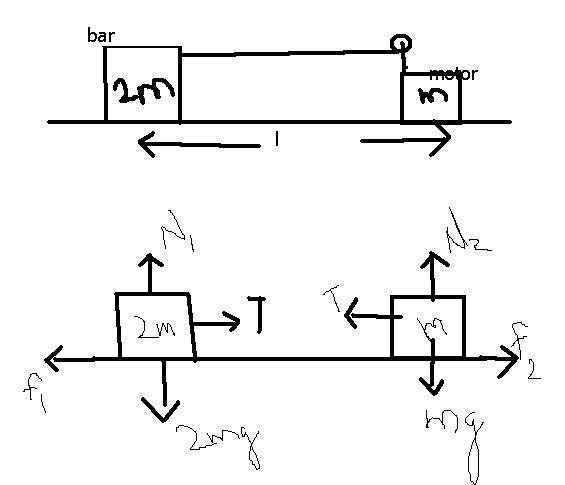
ohk.......lemme ans...
drawing fbd diagrams......
then write all possible dynamics eq..
N1=2mg
f1=kN1=2kmg
therefore
T-f1=2mw1
or T-2kmg=2mw1
according to prob....w1=w
therefore T-2kmg=2mw...............i
N2 =mg
f2 =kmg
T-kmg=mw2....................ii
subtractiing eq ii from i
T-2kmg=2mw
T-kmg=mw2
we get
-kmg=2mw-mw2
w2 =2w +kg
now use kinematical relations
let t be the time after which they collide
s1=distance which bar travelled in time t=12 wt2
s2=distance which motor body travelled in time t=12w2t2
For collision to occur
s1 + s2=l
l=12 wt2 +12 w2t2
putting value of w2 from eq iii ...in above eq we get
t==√2l/(3w+kg)