A sphere is...

VITEEE Discussion Forum › Mechanics questions › A sphere is...

.
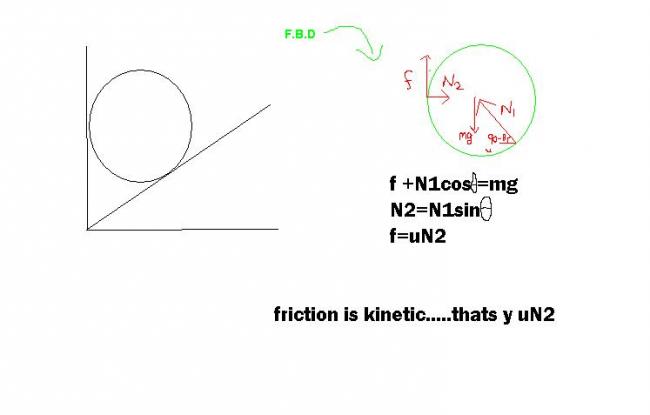
A sphere is rotating between a rough wall and a smooth wedge as shown in the fig. If u is the coefficient of friction between then find the normal reaction between the wall and the sphere.

#Physics #Mechanics

UP 0 DOWN 0 0 7
Post on FacebookPost anonymously?

7 Answers

3

Swastika dutta ·2009-10-07 21:18:15

well.. the answer is not given.

UP 0 DOWN 0
Post on FacebookPost anonymously?

62

Lokesh Verma ·2009-10-07 23:36:08

This is a good problem...

Try to draw the fbd.. that is the most important thing...

Here friction will reduce/ Increase normal reaction... Try to do that.. otherwise I will fill the proof..

UP 0 DOWN 0
Post on FacebookPost anonymously?

1

xYz ·2009-10-07 23:49:10

UP 0 DOWN 0
Post on FacebookPost anonymously?

1

Philip Calvert ·2009-10-08 00:43:39

let the normal reaction from the wall be N and from the wedge be N₁ then

N=N_{1}sin\theta
\mu N+N_{1}cos\theta =mg

\Rightarrow N = \frac{mg}{\mu +cot\theta }

perhaps not so good question unless you move the wedge :P

UP 0 DOWN 0
Post on FacebookPost anonymously?

3

Swastika dutta ·2009-10-08 00:49:05

thanx...:)

UP 0 DOWN 0
Post on FacebookPost anonymously?

62

Lokesh Verma ·2009-10-08 01:12:28

Now in the same problem try and find the number of turns before it stops..

given initial angular velocity is Ï‰₀

UP 0 DOWN 0
Post on FacebookPost anonymously?

3

msp ·2009-10-08 01:27:44

w₀²=2Î±Î¸

find theta and for one revolution theta =2Ï€.

Î±=R(fr₁-fr₂)/I_com

UP 0 DOWN 0
Post on FacebookPost anonymously?

Your Answer

Basic Integral Calculus Operators Symbols Matrix Cases

√ √ || {} [] () → ~ ^ x x x → → ln log lg lim lim cos sin tan cot arcsin arccos arctan

∫ ∫ ∫ ∫ ∫ ∬ ∬ ∬ ∬ ∬ ∭ ∭ ∭ ∭ ∭ ⨌ ⨌ ⨌ ⨌ ⨌

∑ ∑ ∑ ∑ ∑ ∮ ∮ ∮ ∮ ∮ ∏ ∏ ∏ ∏ ∏ ∐ ∐ ∐ ∐ ∐

+ - × ÷ ± ∓ = ∪ ∩ ≠ ∼ ≈ ∅ ∀ ∃ ∈ ∋ ∝ ≤ ≥ ≃ ≅ ≡ < > ≪ ≫ ⊂ ∉ ⊃ ⊆ ⊇ ⇒ ⇐ ⇔ ⇒ ⇐ ⇔ ⇑ ⇓ ⇕ → ← ↔ → ← ↔ ↑ ↓ ↕ ↖ ↗ ↘ ↙ ↪ ↩ ⇀ ↼ ⇁ ↽

° ∂ ∞ α β γ δ θ λ μ ν ξ π ρ σ φ ω ε ƒ κ τ υ Γ Δ Λ Σ Π Ω