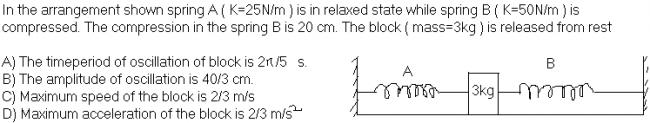
This is a easy question . try it
12 Answers
Yes Ashish, you are right..
Try finding out the Amplitude & max. acceleration of the mass also (and then see the hidden part)
amplitude = 20/3 cm acceleration = 5/3 m/s2
But bhaiya, by simple energy conservation,
12 x (50) x (4) x 10-2 = 12mω2a2
Amplitude = \sqrt{\frac{8}{3}}\times 10^{-1}\: m
where am I going wrong?
During maximum velocity.. that is zero force.. that is equilibrium condition..
there will still be compression in the two springs.. u din consider that I guess!
The initial energy of the system, i.e., when only one spring was stretched and other was not, is conserved. So even at the equilibrium position the energy of the system is equal to the initial energy of the system which again can be equated to 12mω2A2.
Considering what you are saying,
At equilibrium position the total energy of the system is ,
E=\frac{1}{2}K_{1}x^{2}+\frac{1}{2}K_{2}(0.2-x)^{2}+\frac{1}{2}mv^{2}
which is again equal to the initial energy of system. So I guess its not changing anything.
Bhaiya, can you please post your solution.
See..
\small Initial\;energy\;=\;\frac{1}{2}\left(50 \right)\left(\frac{2}{10} \right)^2\\ Final\;energy\;(as\;a\;function\;of\;x)\;=\;\frac{1}{2}\left(50 \right)\left(x \right)^2+\frac{1}{2}\left(25 \right)\left(0.2-x \right)^2+\frac{1}{2}\left(3 \right)\left(v \right)^2\\ \frac{1}{2}\left(50 \right)\left(\frac{2}{10} \right)^2\;=\;\frac{1}{2}\left(50 \right)\left(x \right)^2+\frac{1}{2}\left(25 \right)\left(0.2-x \right)^2+\frac{1}{2}\left(3 \right)\left(v \right)^2\\ or,\;\left(50 \right)\left(\frac{2}{10} \right)^2\;=\;\left(50 \right)\left(x \right)^2+\left(25 \right)\left(0.2-x \right)^2+\left(3 \right)\left(v \right)^2\\
\small or,\;2=50x^2+25x^2+1-10x+3v^2\\ or,\; 3v^2=1+10x-75x^2 for\;v\; to\; be\; min/max,\;3v^2\;will\;be\;min/max\;too..\\ and\;that\;will\;take\;place\;at\; \frac{d}{dx}\left[1+10x-75x^2 \right]=0\\ or,\;10-150x=0\\ or,\;x=\frac{1}{15}
\small at\; x = \frac{1}{15},\;\frac{d^2}{dx^2}\left[1+10x-75x^2 \right]\; is \; negative..\\ i.e.\;x=\frac{1}{15}\; is\; a\; point\; of\; maxima..\\
Or x=115 is the equilibrium position..
The same conclusion could be reached at in a less mathematical way by using concept of equilibrium..
At equilibrium force=0
or, (50)(x)=(25)(0.2-x)
or, 75x=5
or, x=115 which is the same result! [1]
So, amplitude = 0.2m - 115 m = 15m - 115m = 215m = 20015cm=403cm (oops seems my calculations went wrong somewhere in last attempt! :P hadbari me gadbari! :P)
Max. accn. is at extreme position.
accn.=net Forcemass = 50.(0.2)3 = 103m/s2
(hehe! hadbari me itna gadbari ki har ek answer half ho gaya tha!) :P
I might be buggying you now, but either I'm really stupid or I'm imagining stuff!
"(50)(x)=(25)(0.2-x)"
Question: The term (0.2 - x) should be with the spring of spring constant 50N/m right?
I'm really sorry. [2] But somehow, I'm missing something very small.
arey I considered the thing to be at a distance such that there is x compression of 50N/m spring..
u can consider otherwise too.. but then the compression of 50N/m spring will be (0.2-x)