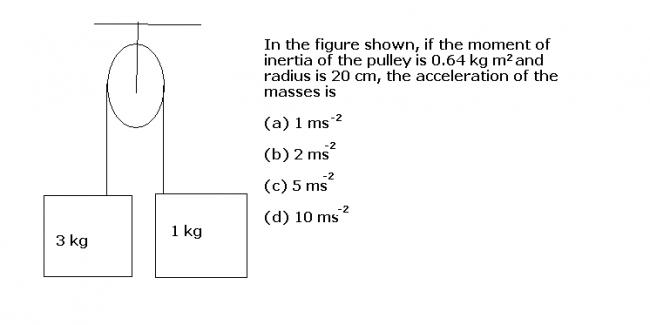
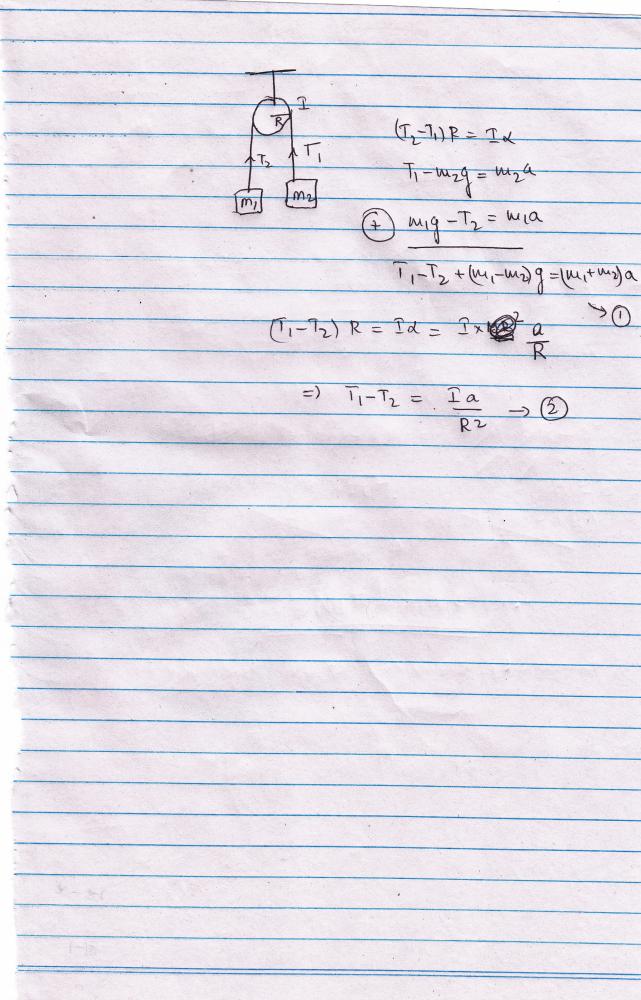IT IS VERY SIMPLE THEN......YOUR OPTIONS R WRONG !!!
A man of mass 'm' stands on a platform of mass 'M' kept on a ice block (friction = 0). If the man walks on the platform with a velocity 'v' w.r.t the platform, the relative velocity of the platform, the relative velocity of the platform w.r.t the ice block is
(a) \frac{mv}{M-m}
(b) \frac{M-m}{mv}
(c) \frac{Mv}{M+m}
(d) \frac{M+m}{Mv}
-
UP 0 DOWN 0 0 13

13 Answers
m(v'+v)+Mv'=0 (i have ignored direction analysis)
=>mv'+mv+Mv'=0
=>v'=-mv/m+M
i gues sthis is a soolvd eg in hcv just check ..... page 153 q 15 .if ma memroy wrks well....
just one doubt: isn't tension in a string same throughout the string???
@Ani: If the tensions are same then the pulley will not rotate. But in this case the pulley rotates and hence T on both sides MUST be different naa to cause the rotation motion of the pulley