is the answer \sqrt{2}-1 ?
A plate, bent at right angles along its center line, is placed onto a horizontal fixed cylinder of radius R as shown in the figure.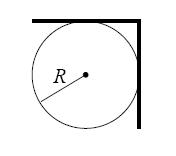
How large does the coefficient of static friction between the cylinder and the plate need to be if the plate is not to slip off the cylinder?
-
UP 0 DOWN 0 3 13

13 Answers
assuming that point of contact is is the midpont of the plates writing friction = K (normal reaction) at both the points of contact . Since we require equilibrium condition we equate all the forces in vertical and horizantal direction for both the bodies and net torque to zero. if k is the coefficient of friction.then finally after solving all the equations we get an equation K2+2K-1=0 since k cannot be neagtive thus K=√2-1. srry could not upload image due to slow net speed
sir is pt of contact mid point of platen parts ????
Que cant be solved without knowing that
Ans follows
i agree celestine... it should be the mid point or else we wont get the ans..
yes...i used the same method as that of celestine's ...sorry cudnt giv the working earlier...
yes celestine your working is right
btw you could have taken the centre of mass and expressed in one mg instead of two mg/2 s..
no rohan that wont be elegant as it makes a short process longer . ( it will take more steps to find torque abt bend pt)
btw i forgot to mention that torque is eq abt bend pt
