absolutely......
A particle moves from rest at A on the surface of a smooth circular cylinder of radius R .At B it leaves the cylinder.The equation relating alpha and beta is??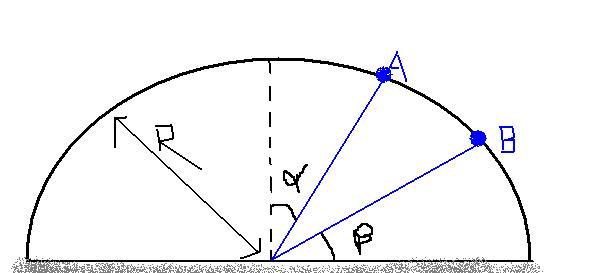
-
UP 0 DOWN 0 1 6

6 Answers
Small Mistake in this one..
this is not very tough eureka...
I will try to solve it..
change of potential energy is
mgR(cos α - cos β) = 1/2 mv2
2g(cos α - cos β) = v2/R
draw fbd at B you will get
v2/R = g sin β
hence 2g(cos α - cos β)=g sin β
2(cos α - cos β)= sin β
See if i have made any mistake.. or let me know if i am right :)
thaanx for help........but ur answer is wrong....u made a mistake here
mgR(cos α - cos β) = 1/2 mv2...........
rather it is mgR(cos α - sin β) = 1/2 mv2
anyways i got the answer........
yeah i did eureka..
thanks for correcting...
I will post the whole solution again :)
this is not very tough eureka...
I will try to solve it..
change of potential energy is
mgR(cos α - sin β) = 1/2 mv2
2g(cos α - sin β) = v2/R
draw fbd at B you will get
v2/R = g sin β
hence 2g(cos α - sin β)=g sin β
2 cos α= 3 sin β
Is this free of errors now?