23btw i learnt the following way of finding IAOR....
take 2 convenient points A and B of a rigid body ........
draw their velocity vectors..........
now draw lines _|_ to the vectors vA and vB
such that the _|_ passes thru the tails of d vectors
the point where these 2 _|_ intersect is the IAOR....
if they dont intersect ...the point is at infinity ....
IS DIS METHOD CORRECT??? ALSO IS DER ANE ODER APPROACH????
a book named vector mechanics and dynamics says that this IAOR cannot be used to fidn acceleration ...y so ?
1not as challenging physics as maths in your question arshad... but I assure you its doable
I have already done that so .... I will let someone else do it
however if no one does I'll just post the answer (and probably some equations)
23
qwerty
·2009-10-06 10:10:15
hey karna pls explain how did u get that answer .......
i guess u took an axis about wich the COM performs pure rotation...so how do we come to kno wer is dat axis ...secondly ..the axis u hav taken ...is the distance of COM always constant from the axis ?how ?? diagram dekh ke lag to nahi raha ???do we need the distance of COM frm axis to be constant ??????
and i m able to understand nothing frm ur one line answers ..pls elaborate [2]
23
qwerty
·2009-10-07 10:50:40
help me out with this one ................. pls some one post a solution that i can understand!!!! PLZ!!! [2]
1qwerty,d axis xyz hs taken is known as d "instantaneous axis of rotation"...u will get to read of it in most d buks...
1.....the normal force at that instant.............
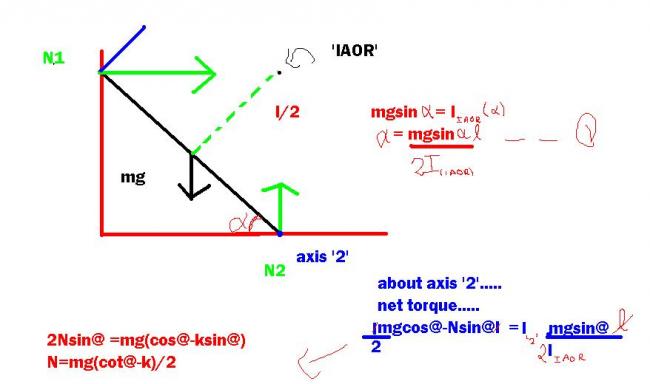
23
qwerty
·2009-10-16 21:00:57
i learnt frm some where that IAOR can be used to find only velocities ..and not accelerations ...is it true??
1i would like to add a question-a very challenging one
find the normal reaction acting at the top end of the rod at this instant.....!!!
lets see who does this first!!!
1
Philip Calvert
·2009-10-17 08:27:46
aah !
qwerty is actually correct (partially) :
"i learnt frm some where that IAOR can be used to find only velocities ..and not accelerations ...is it true"
However the method applied (finding α using torque about IAOR) by xyz is also correct
how can both not be wrong ?? ;)
1
Philip Calvert
·2009-10-17 08:30:19
and if anyone heeded to Nishant bhaiyya's hint ..it would be possible without involving all this IAOR and stuff
1actually this sum cna be solved withouth rotational mechanix if i ma not wrong we hv to use constraint eq ..... i am thinking over it......
23
qwerty
·2009-10-17 09:21:40
but is it possible to simplify the motion in such a way in every problem ???
1
varun.tinkle
·2009-10-17 09:36:30
solved it .....
using constraint hoping u know the methods
first draw a line parallel to the perpendicular and from the com got it..
now consider the groud as a reference for x1 and x2(u know it)
since x1/x2=1/2 midpnt theorem ...
v1/v2=1/2 or vcom in y direction is v/2
for vel in x axis............
first lets find the vel of the other pnt.....
y^2+x^2=l^2
differentiating it
2xvx=-2yvy
solving it we get vel in x direction as vtan@
now for com do the same thing but now take x1 and x2 from aong the perpendicualr since x1/x2=1/2 similarly vx =vtan@/2
now v=v^2x+v^2y
using it we get v^2(1+tan^2)/4 solving it vel is vsec@/2
1
xYz
·2009-10-17 09:38:20
qwerty....no need to worry about this "IAOR"...its just a problem solving technique......but i wud like to add few more things to your post #34
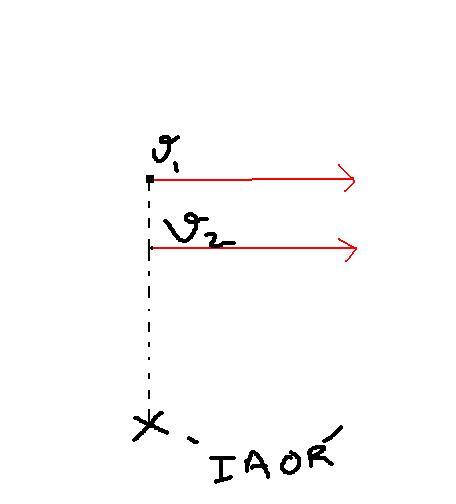
for example......
1
xYz
·2009-10-06 03:41:53
d=l/2....i think....
vcm=2vcosA
23
qwerty
·2009-10-06 03:49:00
as far as i remember ....the ans is v/2 cosecA
or v/2 sec A ..... dont remember exactly
23
qwerty
·2009-10-06 03:49:44
but prof said that u can do it widout rotation also....
1
xYz
·2009-10-06 03:50:28
nishant sir plz tell wer i am wrong...
62This one is often repeated but a very good question...
Hint:
velocity of any point = vcm + velocity due to rotation
Then try...
23
qwerty
·2009-10-06 03:52:47
nishant sir i also hav one more question ...wat will be the path of COM
62
Lokesh Verma
·2009-10-06 03:53:50
path ofthe COM is not very difficult mathemtatically...
But one more thing... I forgot to add...
Think of the Relative velocity fo the rod along the rod,,, what will that be?
62
Lokesh Verma
·2009-10-06 03:54:00
path ofthe COM is not very difficult mathemtatically...
But one more thing... I forgot to add...
Think of the Relative velocity fo the rod along the rod,,, what will that be?
1
xYz
·2009-10-06 03:38:13
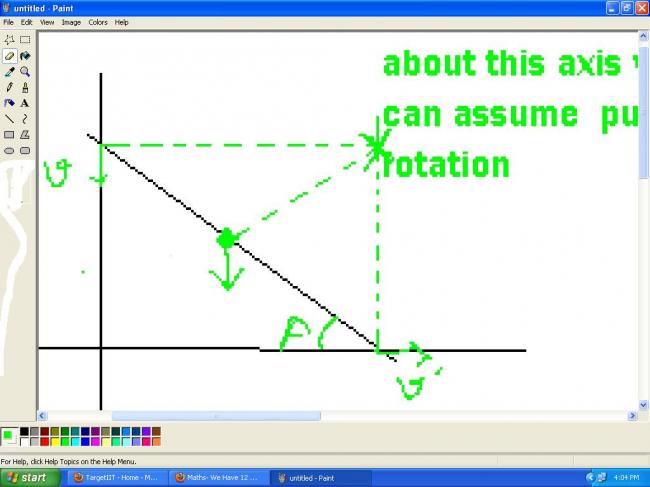
find that distance(b/w axis and com) using geometry
vcm*d=vcosA*l
1
xYz
·2009-10-06 04:38:27
nishant sir about that axis it is pure rotation so....v*l cosA=vcm*l/2......i am quite sure my answer is right......
3@xyz i have a dbt in ur eqn,y u have assumed dat the direction of Vcom is along the y axis
