fluid mechs

i htnk it was done by sharman some time back..

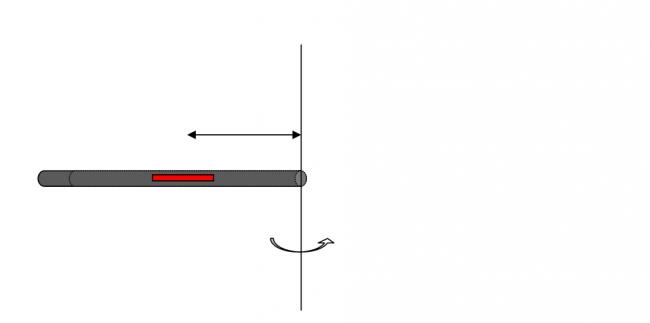
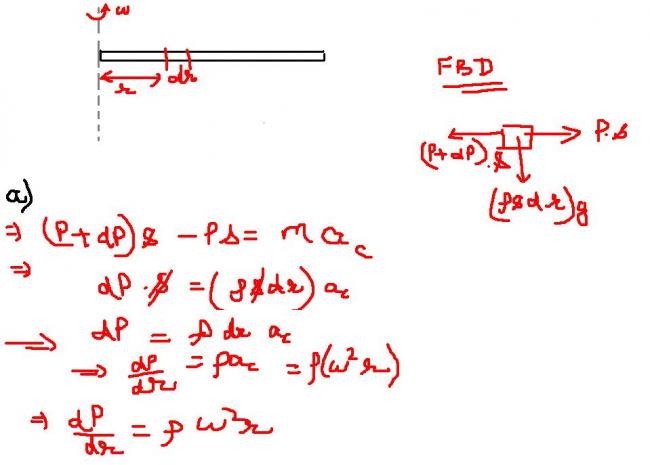Q2. An incompressible fluid with density ρ is in a horizontal test tube of inner cross-sectional area A. The test tube spins in a horizontal circle in an ultracentrifuge at an angular speed ω. Gravitational forces are negligible. Consider a volume element of the fluid of area A and thickness dr' at a distance r' from the rotation axis. The pressure on its inner surface is p and outer surface is p+dp.
(a) Show that dp=ρω²r'dr'.
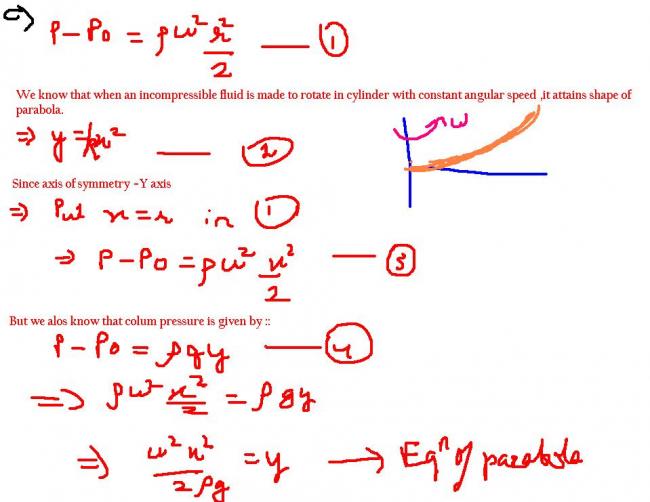
(b) If the surface of the fluid is at a radius r_o where the pressure is p_o, show that the pressure p at a distance r≥r_o is p=p_o+ρω²(r²-r_o²)/2.
(c) An object of volume V and density ρ_ob has its centre of mass at a distance R_cmob from the axis. Show that the net horizontal force on the object is ρVω²R_cm, where R_cm is the distance from the axis to the center of mass of the displaced fluid.
(d) Explain why the object will move inward if ρR_cm>ρ_obR_cmob and outward if ρR_cm<ρ_obR_cmob.
(e) For small objects of uniform density, R_cm = R_cmob. What happens to a mixture of small objects of this kind with different densities in an unltracentrifuge?

Please give a diagram along with answer as well. I cudnt do this quesn bcz cudnt understand the diag.

Q1

i have done simple derivative...u can take it as partial derivative..it wont have an effect on overall eqn[1]

assume a tube and take an elment dr at distance r from axis

Q2 is also same...i hope u can solve it now[1]

i too didnt get those terms dude....[2]
thats why didnt reply....

yes sir i was able to solve the first two bits.. but then Rcm, Rcmob .. i cudnt understand their meanings and positions ...