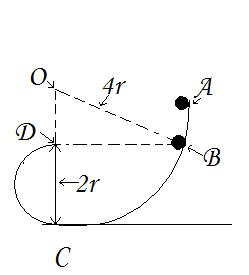A loon the loop apparatus is made of quadrant of radius 4r joined to semi circle of radius r as shown. the track is frictionless and a small particle of mass m slides down starting from the highest point A of the quadrant. calculate the speed, tangential and normal accelerations of the particle at B. with what velocity does it leave the track at D?
1B:
12mv2=mg(4r)-mg(2r)
vB=2√gr
Centripetal accelration=v2r=4g
D:
12mv2=mg(4r)-mg(2r)
vD=2√gr
1corrections in above prob
as Vb=2rootgr which is right
ac=v2/4r=g
at=groot3/2
velocity at D is correctly done
39Akshay it's much easier doing it by energy conservation and then applying v2 = u2 + 2as for tangential acc, don't you think? u = 0, v = vB, s can be approximated to be 2r(the distance AB).
4gr = 2 . 2r . aT
aT = g. There is no other force which is acting on the particle to try and bring it out of its circular motion other than gravity either.
1first of all the equation of motion cannot be applied as it is only applicable to constant acceleration motion,which is not the case here
secondly,a tangential acceleration only depends on net tangential force,Normal force has 0 component along tangential,but Weight will have component as shown in the figure which is only responsible for that instantaneous tangential acceleration.
Hope u got it