The question's done but first assure if the wedge is fixed or not..........bye for now...rath's tuition ...no time to post soln.......i can post it tonight.
 .
.
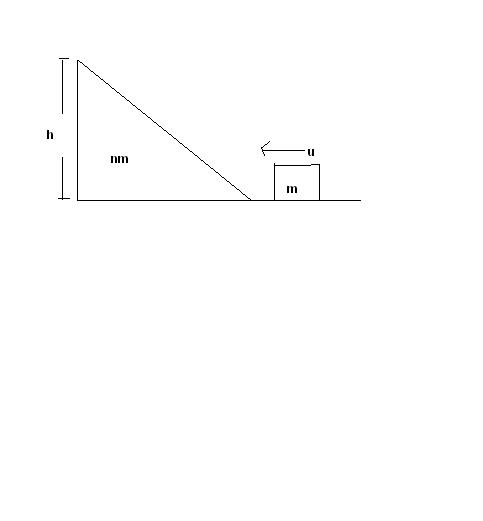
A block of mass m is pushed towards a movable wedge of mass nm and height h with a velocity u. All surfaces are smooth. The minimum value of u for which the block will reach the top of the wedge is...
a> √2gh
b> n√2gh
c> √2gh(1+1/n)
d> √2gh(1-1/n)
-
UP 0 DOWN 0 0 10

10 Answers
First use conservation of linear momentum...
During this period mu=(M+m)V
you get the final v in terms of u (v here is in the horizontal direction)
Now conserve energy.. to take mgh+ 1/2 (m+M) (v2) = 1/2 m u2
Can you solve now?
I think my procedure is correct.But ask Nishant bhaiya to check my ans.....
here is the ans..:
let v is the final velocity of the system..
mu =( m+nm) v
v= u/(1+n).
1/2 u2= 1/2 (1+n) v2+ gh
now put the value v=u/(1+n)
then,
u2=(1+1n)2gh.
hence opt C is correct..
then please post it here so that the thread can be closed swastika [1]
Considering nm=M
mu=(m+M)v
therefore, v=mu/M+m.................(1)
1/2(m+M)v2 +mgh=1/2(mu2).............(2)
putting (1) in(2)
1/2mu2-1/2{(mu)2}/(M+m) = mgh
Solving it...by replacing M=nm.
u2(1-[m/(nm+m)])=2gh
furthur solving it.
I am getting opt c is correct.