Sorry ....But i didnt get what u are trying to say and i couldnt conclude anything from the derivation as well!!!![2]
In work energy theorem we say that the net work done on the body = net change in the kinetic energy of the body.....
why, here, do we involve the kinetic energy only... and why is it not potential energy or mechanical energy????
It may be a silly doubt [7]..... but please try to answer!!!![1]
-
UP 0 DOWN 0 0 13

13 Answers
This is a very good doubt..
See the work done by gravity is -ve of grav potential energy
So if you see closely,
net work done on the body = net change in the kinetic energy of the body
work done on the body by gravitaion + by other forces = net change in the kinetic energy of the body
Thus,
-ve of gravitational potential energy + by other forces = net change in the kinetic energy of the body
Thus work by all forces other than gravitation = net change in kinetic + potential energy...
For a even better understanding try to understand the derivation of work energy theorem (it is a one line derivation :)
ok...
I will give the derivation of work energy theorem
F.ds = dW
For all the external forces,
\sum{F_i} = m.a
Thus,
\sum{F_i} = m.\frac{dv}{dt}
\sum{F_i.ds} = m.\frac{dv}{dt}.ds
\sum{F_i.ds} = m.\frac{ds}{dt}.dv
\sum{F_i.ds} = m.v.dv
Integrating on both sides
\int \sum{F_i.ds} = \int m.v.dv
\sum{\int F_i.ds} = \int m.v.dv
\int F_i.ds is the work by each individual force
\int m.v.dv = 1/2m(v_f^2-v_i^2)
now see the LHS has mg as force... so the potential energy is taken care by the work done by graviation on the LHS..
That is why we only consider kinetic energy..
ok.....
u mean to say that when we integrate f.ds then it is the total work done and hence it means that it includes the work done by gravity as well, and thus includes the potential energy...... and hence obviously we wont add it again.....
is this the correct interpretation?????
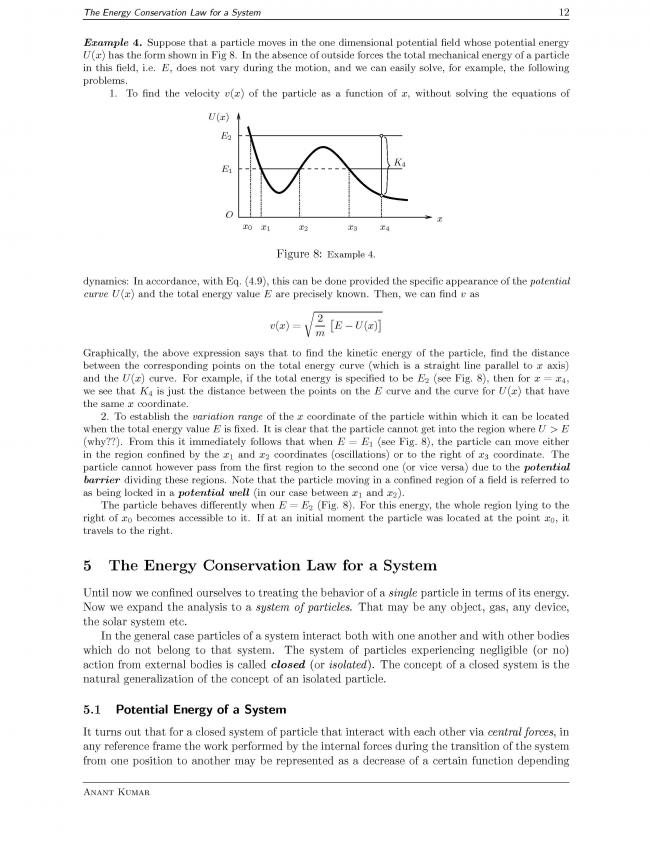
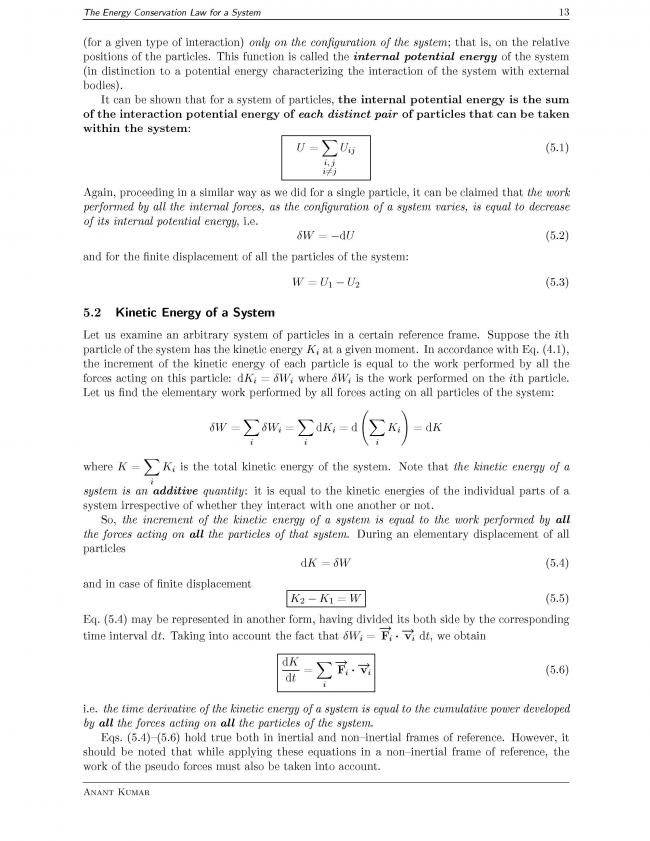
One more thing. What Nishant Sir did with the gravitational force can be done for ANY conservative force. I thought that I'll upload a few pages of my own (yet to be published) book's manuscript. Hope it is useful.




brilliant stuff sir ... tellme when ur buk is releasing will be the first 1 to buy it ....[1][1]
I am trying my best to have it published by the end of this year. Let's see if I succeed or not.