66an additional constraint is required: the stationary one has to be lighter and the collision must be perfectly elastic.
prove that...
If a ball of mass m collides with another stationary ball of mass M then the maximum angle of deviation of the ball of mass m is sin-1M/m
...from post (http://targetiit.com/iit_jee_forum/posts/balls_in_a_semi_circle_3624.html )
-
UP 0 DOWN 0 5 10
10 Answers
66
33cool!!
now what if M>m? does some angle of max deviation exist?
This is much more easier than for M<m..
above question was not Phy... but M>m is physics ;)
9if M>m
of course angle of max deviation is 180
eg a light ball rebounding on a heavy ball
66This problem has been done... however, it is said that a problem has more than one solution. In this case, there are at least two more ways to solve the problem. Can you guys try?
1let me try my brainz
proceeding as ashish... we kbnow v1 and the cmponents
so resultant vel v is vnormal2+valong2
we kknow v normal as v1sin@ and v along as 2m/m+M vcos@
now sin@1=reultant vel v/initial vel v
for maximum value we hv to differentiate it is this correct kaymant sir
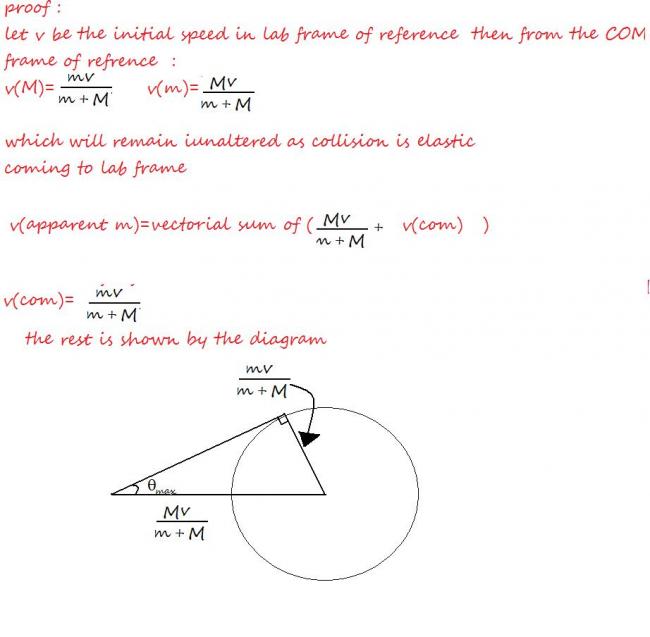
1well today i saw the proof of this lemma
they proved it superbly (but i will prefer asish's way )