hmmmm fine
I C or Instantaneous Centre of Rotation
It is a simple theory to make problems of specific type easier.........if wisely used.....
It states When a body is moving on horizontal floor with pure rolling, then it is as good as the body rotating with the point of rotation as the centre of rotation.
The centre so considered is called instantaneous centre of rotation.

the theory states also to find out the respective instantaneous centres of rotation....
it says the instantaneous centre of rotation of any body lies on the perpendicular on the linear velocity vector of the body.
in the above figure, this can be shown thus......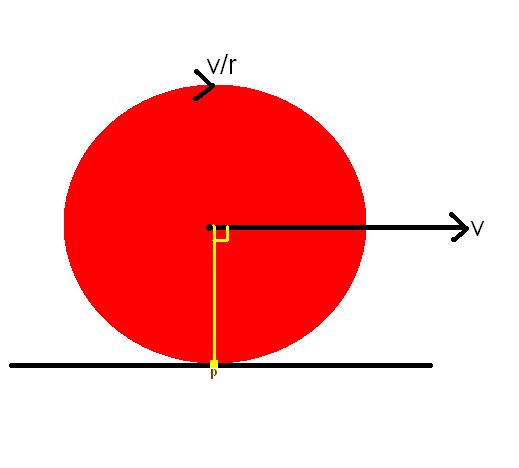
the point p lies on the perpendicular to velocity vector.....
this thread continues...i hope so.....
-
UP 0 DOWN 0 8 53

53 Answers
lets see a question.......
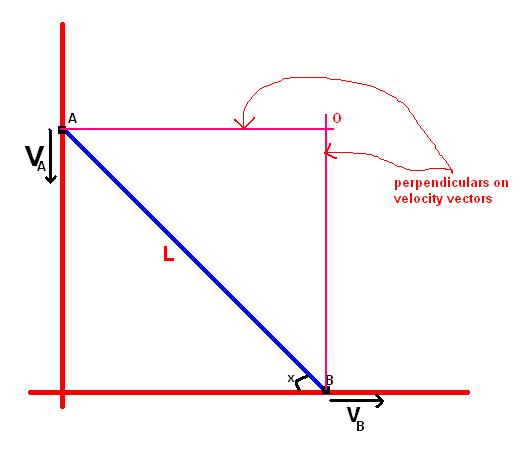
the problem tells that a ladder of length L is resting on a wall making an angle x with horizontal ground.........we have to find angular velocity of the ladder. also find relation b/w vaand vb.
let OB=r.
then using IC ω=Vb/r=Vb/Lsinx.
let B=(b,0) and A=(0,a)
OB=b and OA=a
now a2+b2=L2
differentiating both sides with respect to time,
2a\frac{\partial a}{\partial t}+2b\frac{\partial b}{\partial t}=0
or,2aVa+2bVb=0
or, aVa+bVb=0
or, a/bVa+Vb=0
or, Vb+tanxVa=0
good problem......if possible provide more info and everyone please continue this thread
tho not IC it is good.......

we hav to find tension T when one of the strings just snaps
only me is onl9????????sab so gaye itna jaldi?????????
chalo i am going to post my solution to the above problem hoping it to be correct.......n den i will upload questions for tomorrows 1st hour strife..........
Γ=Iα,
mg.L/2=1/3mL2.a/(L/2)
or, a=3g/4
now, mg-T=ma
or, mg-T=3mg/4
or, T=mg/4
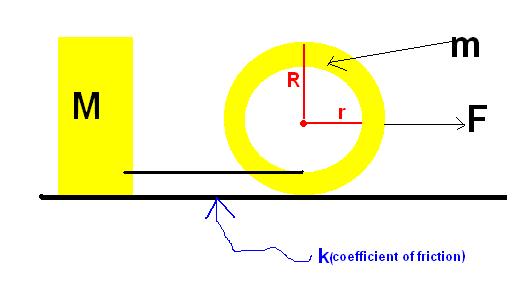
ring has mass m and block has mass M.Find acceleration of the block, the ring the angular accn of ring and the Tension in the string.
P.S. the force F passes through c.m. of ring....
similar probs....
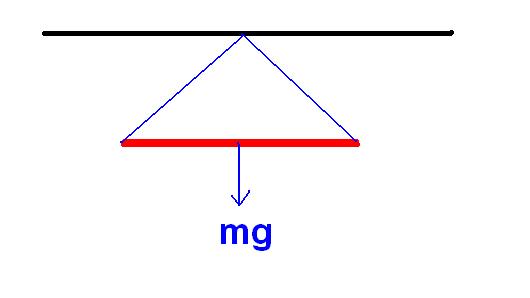
1.)
2.)
for both the problems, mass of rod is m, length is L and the triangle and trapezium formed are isoceles.......
we have to find the tension in one of the strings just at the momment the other snaps.
problem on variable torque and variable moment of inertia......
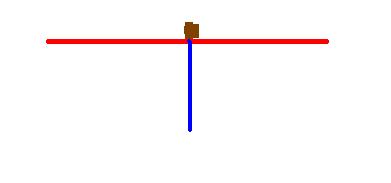
a uniform rod(red) of length L and mass m is pivoted to horizontal ground at its mid point.an insect of mass equal to the rod(brown mark)hey it must be really large one falls on the mid point of the rod. afte sometime it feels tired to sit at one fixed point and starts moving towards one of the ends of the rod..........find angular accn of the rod as a function of x(the dist of the insect from the mid point of the rod).

both the bodies initially rolling purely undergo perfectly elastic collision.
find their velocities when they again start pure rolling after the collision...
this is my last one for today......

the rotating ball has moment of inertia=I, initial angular vel=ω,
linear vel. just before hitting the plank=v.
given coefficient of restitution(e)=1/2
the plank and ground has no friction but the upper surface of plank has friction.
given that the ball stops rotating due to the collision, find the distance moved by the block on the plank between the interval of its first collision with planck and second collision.
P.S. the coefficient of friction b/w the plank and sphere is μ.
HINT:make use of impulse
P.S.surely this is the most complex sum of the night......i challenge.....in mechanics at least.....it had taken me 4 days to solve.....
well et me try my hand
first let the rotational impulse imparted by the plank x then the same impulse will be imparted to the plank but here it will become linear impulse
first since the vel is o thereofre impuse is chang in angular momentum which is equa to (mvr+iw-mvr/2)
here angular impulse is Jr
now the linear impuse on the plank will be J which is equal to thte change in its momentum
we know the vel of the sphere as well as the vel of the plank then we can easily calculate the distance moved
P.S. i think i hv made a mistake as there was no need for the coefficient of restitiution in the whole sum
the sphhere sum is the easiest first the interacting forces will result in ttwo types of impulses one linear impulse and the other angualr impulse...
the linear impulse is ineffected by the prpperties of the rolling effects
so simply using the principle and the formulas for elastic collision
we know the vel of the respective spheres
supposuse the vel of 1 is v1 and the other v2
...........
this is the simpler parts
fro the time instants of the collision the impulse is 0 so during the collisions angular vel will not change
now its very easy tto find the final vel
we can use angualr momentum or linear acc method
btw i had a doubt in IAOR ....i read in a book that IAOR can be used only to find velocities...and accelerations cant be found out using it ....y is it so?
but i thought differently........
e=vel of recoil/vel of approach.
thus in vertical direction,
vel after collision v1=v/2
let an impulsive normal force N act on the bodies in time dt.......
Ndt=change in momentum=3mv/2........(i)
now, there would be friction acting on the sphere due to impulsive normal reaction in right direction. f=μN......(ii)
now this provides an anticlockwise impulcive torque.....
now, impulsive torque*dt=change in angular momentum
or, μNdt=Iω-I*0=Iω
or, from (i), Iω=μ3mv/2........(ii)
now the frictional force would give a horizontal force on sphere,
thus, μNdt=mvhorizontal
or, mvhorizontal=μ3mv/2
or, vhorizontal=3μv/2.......(iii)
also the friction will act on the planck in left direction.....
thus, μNdt=Mvplanck
or, Mvplancl=μ3mv/2........(iv)
next approach for projectile motion.......
[1] [1] [1]
For #11... what do we take the MOI as ??
Ml2/12 or ml2/3 why??
For #13 is there friction between the two colliding spheres?
@ ashis....we take 1/3 ml2
it is from parallel axis theorem.....n also as axis of rotation is not through md.pt.
no hw can there b friction b/w the two spheres, there is no elative motion only......bt there is surely friction b/w the floor and the balls........
i take the previledge to ask another simple question over here......
a sphere is rolling on a surface, mass is m, μ is coeff of fric.
what is the value of friction acting???????????
P.S. there may or may not be pure rolling.......
shall i complete my solution in #19??????????????
btw every one see http://targetiit.com/iit-jee-forum/posts/p-c-12432.html
where was i wrong???????????