1the velocity of b wrt a is
vr=(va2+vb2+2.va.vbcosδ)1/2
where δ=Π+θa-θb
so ωb wrt a =vr/rsinθ
θ is the angle btw vr & x-axis
12 Answers
1
3yes aragorn whn u r in the frame of reference of A or B the other particle will seems to rotate
1priyam, i think ur ans is right...this was one of the options given..
but hw did u do it??
1yes priyam's ans correct..
take the perpendicular components of vel...
then relative ang speed= rel linear speed/ displacement between the two points..
1similoar case..
jus here u have the vel at some angle...
[remember.... v = r X ω ... thats y we will take the perpendicular component ]
33See...
As velocity (as told beautifully by Sky above (hehehe up above there is sky only :P)) is cross product of r and ω so we only care for velocity perpendicular to r (as it perpendicular velocity is product of ω and r) so only perpendicular component of velocity is shown in teh figure below:
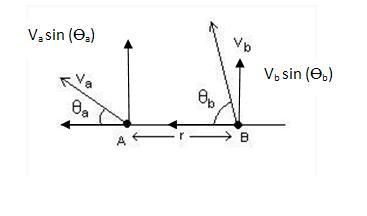
as A is the observer so he (she) sees himself (herself) at rest and adds his (her) negative velocity to other particle (here B)..
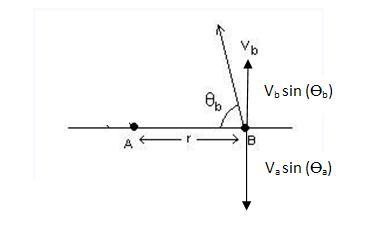
so now wrt A velocity (perpendicular to the distance i.e. r) of B is
(vbsin(θb)-vasin(θa)
so (as it is clear from the elegant example by sky above )
ω is (vbsin(θb)-vasin(θa)
r
Note: don't consider it as division or cross division of vectors..
:P
Just kiddin..
[1]