24
Q) the uniform rectangular (laminar) block in the figure has mass M and dimensions [a,a/2]
which is supported by two massless inelastic strings of equal length whose other ends are attached to a rigid ceiling
Find the angular acceleration (α) and the acceleration of the center of mass (acm) immediately after one of the strings is cut.
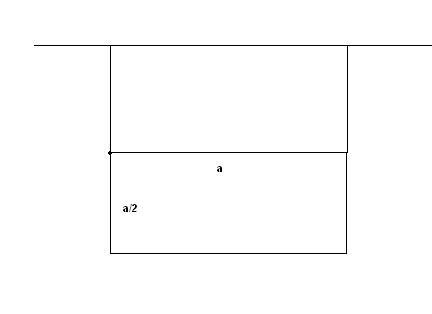
edit : removed everything except the question to make it a neat and tidy reading :)
-
UP 0 DOWN 0 2 48
48 Answers
24are these eqns OK ?????
I will eliminate T after thsi and put the value of I
plz confirm someone
1@eureka why cant v consider pure rotation about the other point where the thread is uncut>????
24@philip
Since u have asked angular accelration and accelration(COM) just after string is cut...point P will remain fixed at that instant
1ok I think now it is time to put things in perspective . Consider all the three situations shown below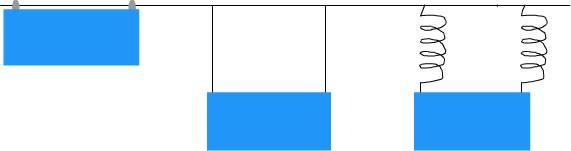
now according to what you all are saying initial values of a and α will be the same for all three [11]
The question to be answered still remains the 2nd situation one only..
but if you are getting no clue to the difference (or simiilarity) then i suggest you try the 1st and 3rd ..which are actually two liners..
PS : the 1st one shows the block hinged at its corners and the third one has identical springs(though they dont quite look like springs) attached to the corners
3@eure even i thot of the same bcos i have seen a similar problem in gmp.Neways i have a dbt in ur eqns,The com is rotating abt the point P.so if we need angular acceleration we can write eqn with respect to point P.Since the com is in rotation motion angular acceleration =acom/dist
1i dont think p will be at rest...p point will also oscillate...wont it ,philip?
1There are about 8 - 10 posts that suggest that point P is going to remain at rest.(or even be the instantaneous axis of rotation !)
Then I dont understand why is no one daring to prove the same
24EIther I am too confused or is philip......
philip in ur question all the values are asked immediately after cutting...
not soem time after cutting....
so do u expect CM to come down in "dt" time interval ?????
1mna no need for dt just apply torque equations get angular accc.. and a=r@ r from the pnt of rotation
1around 430 views but only 25 trys
so i will post the solution now
First very important but simple thing is the use of the equations:
F_{ext}=ma_{cm}
\tau_{c}=I_c\alpha
these are probably the first eqns to appear in their respective chapters ...but some of us seem to have forgotten them or were simply unaware..
the situation just after cutting is shown below: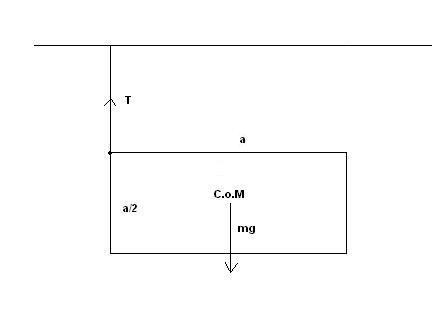
Thus the centre of mass clearly acquires a downward velocity, proving that all of your claims about the IAOR being at the attached end wrong..
now question is almost done ...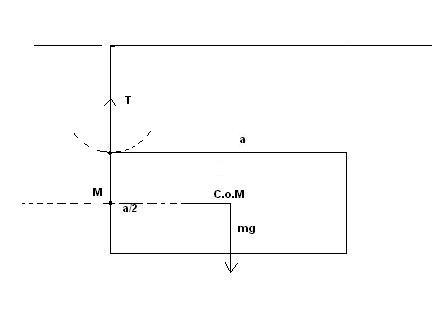
we just proved that the unattached end is not the IAOR so it must be moving.Now comes the easiest part , no matter which way that point moves it is constrained ; so we can locate the IAOR finally which is M ...
now just apply
\tau_{M}=I_M\alpha
