1I didnt specify it as a reason for removing energy ...
energy is always translational + rotational
Q1. A small spherical ball of mass m and radius r rolls in a concave surface of radius R with sufficient friction. Find the time period of the resulting SHM.
Q2. A body of mass m and specific gravity d(>1) floats in water. The temperature of both the body and the water is increased by T. The coefficient of linear expansion of the body is α and volume expansion of water is γ .
(i) Find the initial time period.
(ii) What is the new fraction of the body that is immersed in water at equilibrium
(iii) What is the new time period in terms of the original time period?
-
UP 0 DOWN 0 3 39
39 Answers
1
33Q. 1.267 of erodov..
http://targetiit.com/iit_jee_forum/posts/30th_november_2008_762.html
33Q.1
KE=(1/2)[(2/5)mr2+m(R-r)2]ω12+(1/2)[(2/5)mr2+mr2]ω22
ω1=v/(R-r)
ω2=v/r
dθ/dt=ω1
and then write everything in terms of a and θ/(R-r)=x
...
[12] well maybe...
106[5][5][7][7] dimaag ke upar se flow kar gayaa....
plz thoda aur explanation de sekte ho.. priyam bhai... but i shudnt ask much .... cuz ur an emperor and emperors are known to be ruthless [3]
1v=rw
w' = angular velocity of COM of sphere
w'=v/(R-r)= rw/R-r
dw'/dt=r/R-r dw/dt
alpha'=ralpha/R-r
alpha= a/r
a= gsinx/ 1+I/mr2
a=5gsinx/7
alpha' = 5grsinx/7(R-r)
sinx=x
alpha' = - 5grx/7(R-r)
t=2pi√x/alpha'
T=2pi√7(R-r)/5g
1well dont get confused by apply too much energies
(more energy => less stability)
there will be translational energy and rotational energy = simple..
translational = mv2/2
rotational = Iw2/2=2/5 * mr2w2/2
=mv2/5 (as v=wr for no sliding)
further potential energy=mg(R-(R-r)cosθ)
add them and differentiate u will get
7/5 mvdv/dt=-mgx(dθ/dt)
here dθ/dt = v/R-r
we get
dv/dt=-5mgx/7(R-r)
33http://targetiit.com/iit_jee_forum/posts/30th_november_2008_762.html
isme energy different kyun hai...??
(more energy => less stability) this reason is not enough for removing an energy....
also... i think this is right... because... answer is matching the force method... But How its correct?
106as far as i remember... writing this type of energy was discussed long back...
do u hav the link...
1actually in the question it is mentioned that the mass m is small
thus r<<R which gives that the contribution of velocity by w1 to all the points is almost same ...
as analogous to the case in your link the r is not <<R so the velocity contribution of the w1 there is not same to each point ..
so the case here becomes almost the approaching case of a mass tranlating with v
33But it is not approximation in force method... and answer is same...
but I think i got something... plz see if it can be the reason...
jsut wait for a sec.. don go ofline...
33
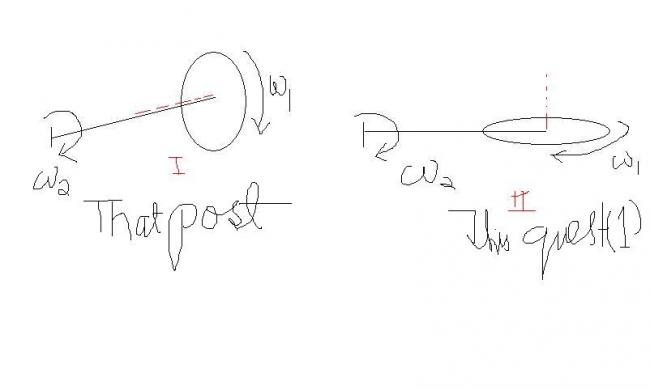
In II (this question).. axis of rot of sphere is not being rotated with ω2 about a fixed point only translated....... so not that energy term
But in I(that link) axis of rot is rotated also.....with ω2 about center as shown.. so that energy term is included in this case...
21iN REFERENCE TO # 29
here dθ/dt = v/R-r
FRM WER DUS THIS CUM ??
33:D
mujhe laga hi tha...
thats why i posted the FBD and then deleted it... bcuase i knew everyone will psot energy method... :D
1v=rw
w' = angular velocity of COM of sphere
w'=v/(R-r)= rw/R-r
dw'/dt=r/R-r dw/dt
alpha'=ralpha/R-r
alpha= a/r
a= gsinx/ 1+I/mr2
a=5gsinx/7
alpha' = 5grsinx/R-r
sinx=x
alpha' = - 5grx/R-r
t=2pi√x/alpha'
T=2pi√(R-r)/5gr
106yes bhaiya ...
this is wat i did..
at any inst.
mv2/2 + Iω2/2 + mg(R-r)(1-cosθ) = const.
==> mω2r2/2 + mr2w2/5 + mg(R-r)(1-cosθ) = const.
==> mωαr2 + 2ωαmr2/5 + mgω(R-r)sinθ = 0
==> αr2(7/5) = -g(R-r)θ
==> α = -(5/7)g(R-r)/r2θ
giving T = 2Ï€r√7/5g(R-r)
but ans is given as 2Ï€√7(R-r)/5g
33mgsinθ-f=ma
fr=(2/5)mr2α
f=(2/5)ma ...a=αr
put sinθ=θ
mgθ-(2/5)ma=ma
mgθ=(7/5)ma
θ=x/(R-r) ...edited... this line :D
a=(5/7)gx/(R-r)
t=2pi√7(R-r)/5g