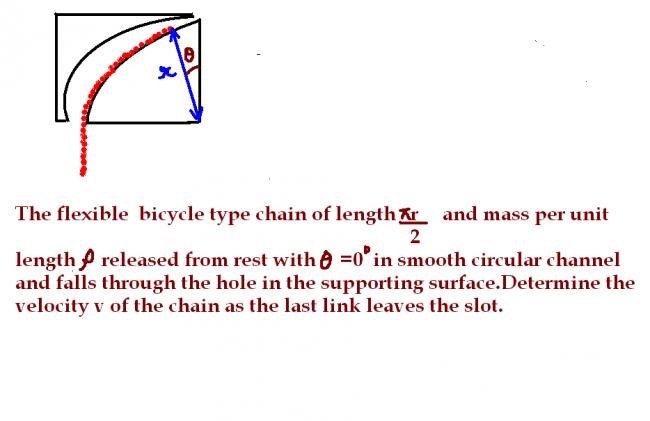
33Is √gR(2/Î +Î /4)
12 Answers
24priyam,,,plz tell the method.....or give an outline of solution.....
1loss in pot energy of com = gain in kinetic energy of com
vel of com= vel of tip of the chian..
33kya???
mine just opposite but how can be this ....
surely answer is wrong in the book :(
:(
:(
someone else plz verify...
I am not posting my method just now as it will make many others to bring my (wrong) answer :(
plz others verify....
24come on priyam ......post ur solution...........the book may be wrong also.......
33Initially COM of the chain(when θ=00) is at 2R/Πabove the base.
Finally (when θ=900) COM is ΠR/4 below base so 1/2mv2=mg(2R/Π+ΠR/4)..
and hence the answer :)
1It is said that the chain starts at θ = 0
So at that position , chain's potential energy is Ïgr^2
Final position , it is having a displacement of - πr/4
So final potential energy = [ -( Ï€^2)(r^2)Ïg ]/8
Hence change is potential energy = kinetic energy
So ,
r^2Ïg(1+Ï€ ^2/8) = 1/2 . (Ï€. r )/2 . Ï.v^2
Solving this we should get the required result ,
v = √rg(Ï€/2+4/Ï€)